Page 40 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY
P. 40
Unit 4: Integration
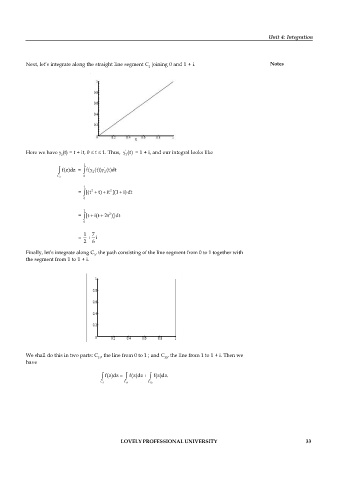
Next, lets integrate along the straight line segment C joining 0 and 1 + i. Notes
2
Here we have (t) = t + it, 0 t 1. Thus, ' 2 (t) = 1 + i, and our integral looks like
2
1
f(z)dz = f( (t)) (t)dt 2 ' 2
C 2 0
1
2
2
= [(t t) it ](1 i) dt
0
1
= [t i(t 2t )]dt 2
0
1 7
= i
2 6
Finally, lets integrate along C , the path consisting of the line segment from 0 to 1 together with
3
the segment from 1 to 1 + i.
We shall do this in two parts: C , the line from 0 to 1 ; and C , the line from 1 to 1 + i. Then we
32
31
have
f(z)dz f(z)dz f(z)dz.
C 3 C 31 C 32
LOVELY PROFESSIONAL UNIVERSITY 33