Page 41 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

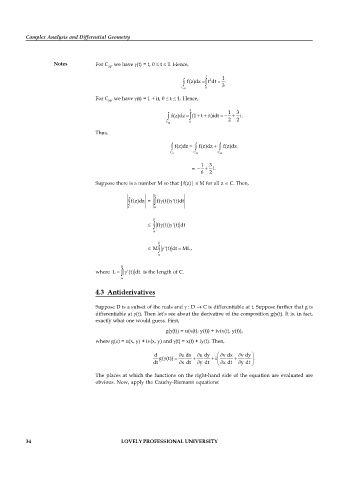
Notes For C , we have (t) = t, 0  t  1. Hence,
31
1 1
2
 f(z)dz   t dt  .
C 31 0 3
For C , we have (t) = 1 + it, 0  t  1. Hence,
32
1 1 3
 f(z)dz   (1 t it)idt    2 i.


2
C 32 0
Thus,
 f(z)dz   f(z)dz   f(z)dz.
C 3 C 31 C 32
1 3
=   i.
6 2
Suppose there is a number M so that |f(z)|  M for all z  C. Then,


 f(z)dz =  f( (t)) '(t)dt


C 


  f( (t)) '(t) dt




 M  '(t) dt  ML,


where L    '(t) dt is the length of C.


4.3 Antiderivatives

Suppose D is a subset of the reals and  : D  C is differentiable at t. Suppose further that g is
differentiable at (t). Then let’s see about the derivative of the composition g((t). It is, in fact,
exactly what one would guess. First,

g((t)) = u(x(t), y(t)) + iv(x(t), y(t)),
where g(z) = u(x, y) + iv(x, y) and (t) = x(t) + iy(t). Then,

d  u dx  u dy   v dx  v dy 

dt g( (t))   x dt   y dt  i    x dt   y dt  . 
The places at which the functions on the right-hand side of the equation are evaluated are
obvious. Now, apply the Cauchy-Riemann equations:

34 LOVELY PROFESSIONAL UNIVERSITY