Page 42 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Unit 4: Integration

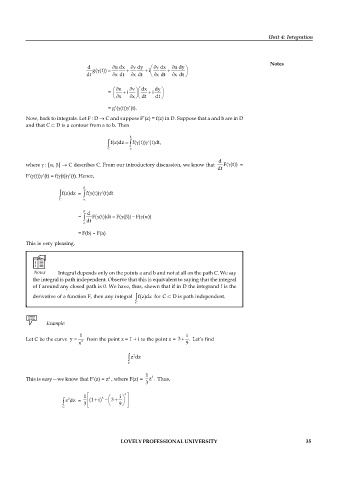
d  u dx  v dy i   v dx  u dy  Notes

dt g( (t))   x dt   x dt     x dt   x dt  

=    u  i  v    dx  i dy  
 x   x  dt dt 

= g’((t))’(t).
Now, back to integrals. Let F : D  C and suppose F’(z) = f(z) in D. Suppose that a and b are in D
and that C  D is a contour from a to b. Then


 f(z)dz   f( (t)) '(t)dt,


C 
d
where  : [, ]  C describes C. From our introductory discussion, we know that F( (t)) =

dt
F’((t))’(t) = f((t))’(t). Hence,


 f(z)dz = f( (t)) '(t)dt


C 
 d
=  F( (t))dt  F( ( )) F( ( ))






 dt
= F(b) – F(a)
This is very pleasing.
Notes Integral depends only on the points a and b and not at all on the path C. We say
the integral is path independent. Observe that this is equivalent to saying that the integral
of f around any closed path is 0. We have, thus, shown that if in D the integrand f is the
derivative of a function F, then any integral f(z)dz for C  D is path independent.

C

Example:
1 i
Let C be the curve y  from the point z = 1 + i to the point z = 3  . Let’s find
x 2 9
2
 z dz
C
1
3
This is easy—we know that F‘(z) = z , where F(z) = z . Thus,
2
3
1  3  i  3 
2
 z dz =  (1 i)   3   

C 3    9   

LOVELY PROFESSIONAL UNIVERSITY 35