Page 106 - DMTH404_STATISTICS
P. 106
Statistics
Notes Alternative Method :
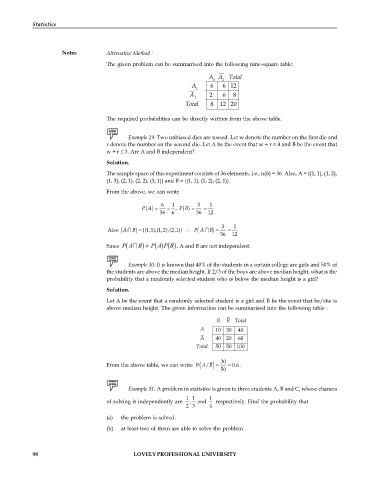
The given problem can be summarised into the following nine-square table:
B B Total
A A
2 2
A A 6 6 12
1
A 1 2 6 8
Total 8 12 20
The required probabilities can be directly written from the above table.
Example 29: Two unbiased dice are tossed. Let w denote the number on the first die and
r denote the number on the second die. Let A be the event that w + r 4 and B be the event that
w + r 3. Are A and B independent?
Solution.
The sample space of this experiment consists of 36 elements, i.e., n(S) = 36. Also, A = {(1, 1), (1, 2),
(1, 3), (2, 1), (2, 2), (3, 1)} and B = {(1, 1), (1, 2), (2, 1)}.
From the above, we can write
6 1 3 1
P A , P B
( )
( )
36 6 36 12
3 1
Also (A B ) {(1,1),(1,2),(2,1)} P (A B )
36 12
Since P A B b g b g
P A P B b g , A and B are not independent.
Example 30: It is known that 40% of the students in a certain college are girls and 50% of
the students are above the median height. If 2/3 of the boys are above median height, what is the
probability that a randomly selected student who is below the median height is a girl?
Solution.
Let A be the event that a randomly selected student is a girl and B be the event that he/she is
above median height. The given information can be summarised into the following table :
B B Total
A 10 30 40
A 40 20 60
Total 50 50 100
)
From the above table, we can write ( /P A B 30 0.6 .
50
Example 31: A problem in statistics is given to three students A, B and C, whose chances
1 1 1
of solving it independently are , and respectively. Find the probability that
2 3 4
(a) the problem is solved.
(b) at least two of them are able to solve the problem.
98 LOVELY PROFESSIONAL UNIVERSITY