Page 193 - DMTH404_STATISTICS

Unit 14: Theoretical Probability Distributions

Notes
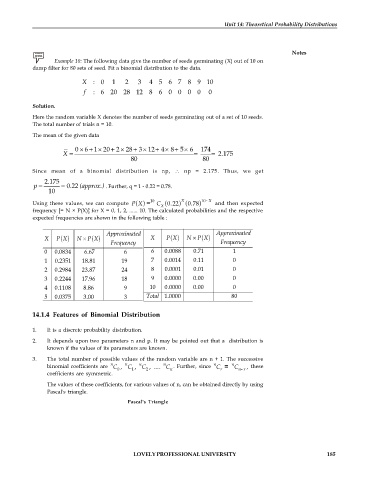
Example 10: The following data give the number of seeds germinating (X) out of 10 on
damp filter for 80 sets of seed. Fit a binomial distribution to the data.
X : 0 1 2 3 4 5 6 7 8 9 10
f : 6 20 28 12 8 6 0 0 0 0 0

Solution.

Here the random variable X denotes the number of seeds germinating out of a set of 10 seeds.
The total number of trials n = 10.
The mean of the given data

´
+
0 6 1 20 2 28 3 12 4 8 5 6 174
´
+
´
+
´
+
´
+
´
X = = = 2.175
80 80
Since mean of a binomial distribution is np,  np = 2.175. Thus, we get
2.175
p = = 0.22 (approx.). Further, q = 1 - 0.22 = 0.78.
10
Using these values, we can compute P X a f = C a 0.22f a 0.78f 10- X
X
10
X and then expected
frequency [= N × P(X)] for X = 0, 1, 2, ...... 10. The calculated probabilities and the respective
expected frequencies are shown in the following table :
Approximated Approximated
( )
( )
( )
( )
X P X N P X X P X N P X
´
´
Frequency Frequency
0 0.0834 6.67 6 6 0.0088 0.71 1
1 0.2351 18.81 19 7 0.0014 0.11 0
2 0.2984 23.87 24 8 0.0001 0.01 0
3 0.2244 17.96 18 9 0.0000 0.00 0
4 0.1108 8.86 9 10 0.0000 0.00 0
5 0.0375 3.00 3 Total 1.0000 80
14.1.4 Features of Binomial Distribution
1. It is a discrete probability distribution.
2. It depends upon two parameters n and p. It may be pointed out that a distribution is
known if the values of its parameters are known.
3. The total number of possible values of the random variable are n + 1. The successive
n n n n n n
binomial coefficients are C , C , C , .... C . Further, since C = C , these
0 1 2 n r n- r
coefficients are symmetric.
The values of these coefficients, for various values of n, can be obtained directly by using
Pascal's triangle.
Pascal's Triangle

LOVELY PROFESSIONAL UNIVERSITY 185