Page 194 - DMTH404_STATISTICS

Statistics

Notes

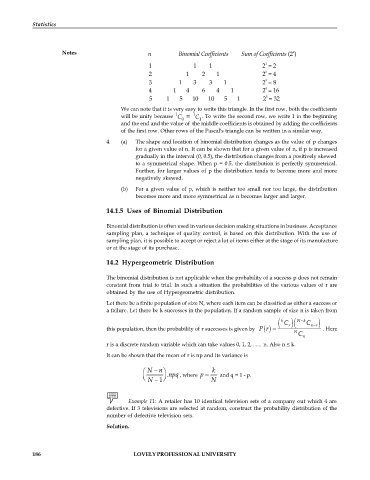
We can note that it is very easy to write this triangle. In the first row, both the coefficients
1
1
will be unity because C = C . To write the second row, we write 1 in the beginning
0 1
and the end and the value of the middle coefficients is obtained by adding the coefficients
of the first row. Other rows of the Pascal's triangle can be written in a similar way.
4. (a) The shape and location of binomial distribution changes as the value of p changes
for a given value of n. It can be shown that for a given value of n, if p is increased
gradually in the interval (0, 0.5), the distribution changes from a positively skewed
to a symmetrical shape. When p = 0.5, the distribution is perfectly symmetrical.
Further, for larger values of p the distribution tends to become more and more
negatively skewed.
(b) For a given value of p, which is neither too small nor too large, the distribution
becomes more and more symmetrical as n becomes larger and larger.

14.1.5 Uses of Binomial Distribution

Binomial distribution is often used in various decision making situations in business. Acceptance
sampling plan, a technique of quality control, is based on this distribution. With the use of
sampling plan, it is possible to accept or reject a lot of items either at the stage of its manufacture
or at the stage of its purchase.

14.2 Hypergeometric Distribution

The binomial distribution is not applicable when the probability of a success p does not remain
constant from trial to trial. In such a situation the probabilities of the various values of r are
obtained by the use of Hypergeometric distribution.
Let there be a finite population of size N, where each item can be classified as either a success or
a failure. Let there be k successes in the population. If a random sample of size n is taken from
( )( N k C n r )
k

C

r
P
this population, then the probability of r successes is given by ( ) r = . Here
N
C
n
r is a discrete random variable which can take values 0, 1, 2, ...... n. Also n  k.
It can be shown that the mean of r is np and its variance is

æ N nö k
ç ÷ .npq , where p = and q = 1 - p.
è N  1 ø N
Example 11: A retailer has 10 identical television sets of a company out which 4 are
defective. If 3 televisions are selected at random, construct the probability distribution of the
number of defective television sets.

Solution.

186 LOVELY PROFESSIONAL UNIVERSITY