Page 283 - DMTH404_STATISTICS

Unit 21: Confidence Intervals

Notes

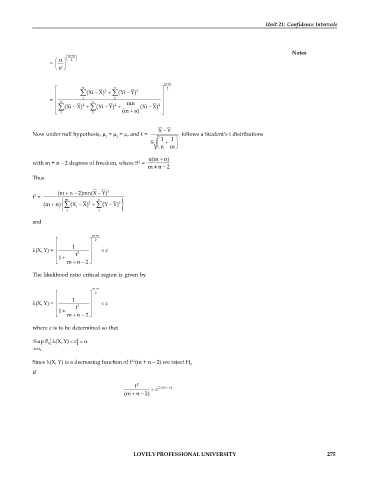
 m n 
 u    2  
=  
 u' 

m n
 m 2 n 2  2


  (Xi X)   (Yi Y) 
=  m 1 n 1 
 2 2 mn 2



  (Xi X)   (Yi Y)  (Xi X) 

 1 1 (m n) 
X Y

Now under null hypothesis,  =  = , and t = follows a Student’s t distributions
1 2
 1 1 
S   
 n m 
u(m  n)
2
with m + n – 2 degrees of freedom, where S =
m  n 2

Thus



(m n 2)mn(X Y) 2
t =
2
 m n 2 
2
(m n)  (X  X)   (Y Y) 


i
 1 1 
and
m n

  2
 1 
(X, Y) =  2   c
 1  t 

  m n 2  

The likelihood ratio critical region is given by

m n
  2
 1 
(X, Y) =  2   c
 1  t 


  m n 2  
where c is to be determined so that
Sup P  (X,Y) c    


0
2/
Since (X, Y) is a decreasing function of t (m + n – 2) we reject H
0
if
t 2 2 /(m n)

 c
(m  n 2)


LOVELY PROFESSIONAL UNIVERSITY 275