Page 288 - DMTH404_STATISTICS

Statistics

Notes n
–1
Let X = n  X . Consider the interval (X a,X b).  In order for this to be a (1 – )-level
i
1
confidence interval, we must have
1

P{X a    X  b}   
Thus

  b (X   ) a  
1
P  n  n  n   


    b  
(X   )
Since, n /~ N(0,1) we can choose a and b to satisfy

  b (X   ) n a  
P  n   n  = 1 – a

      
provided that a is known. There are infinitely many such pairs of values (a, b). In Inference
particular, an intuitively reasonable choice is a = b = c , say
In that case

c n
 Z /2 where Z is the /2 percent point of the standard normal distribution, and the
  /2
confidence interval is

(X ( / n)Z ,X ( / n)Z )




 /2  /2
The length of the interval is (2 / n) Z  /2 Given a and a one can choose n to get a confidence
interval of desired length.
2
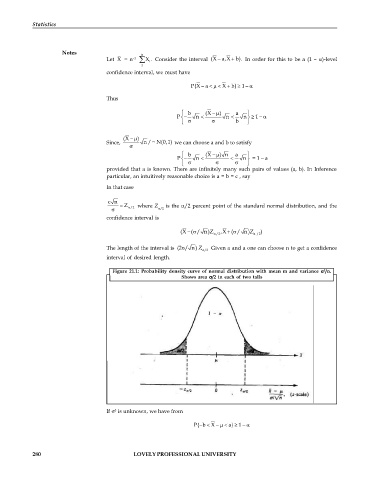
Figure 21.1: Probability density curve of normal distribution with mean m and variance  /n.
Shows area /2 in each of two talls

If  is unknown, we have from

1

P{ b  X    a}   

280 LOVELY PROFESSIONAL UNIVERSITY