Page 373 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Differential and Integral Equation

Notes u ( ) = u 10 at r a
r
r
and u ( ) = u 20 at r b
22.2.2 Steady Flow of Heat in Rectangular Plate

We now consider the steady state temperature distribution in a rectangular metallic sheet. In
this case temperature is every where independent of time, and hence the equation governing the
temperature distribution is given by

2 2
V V
= 0 ...(i)
x 2 y 2
This equation is called Laplace s equation of two Dimensions. We shall now solve this equation
under various boundary conditions.

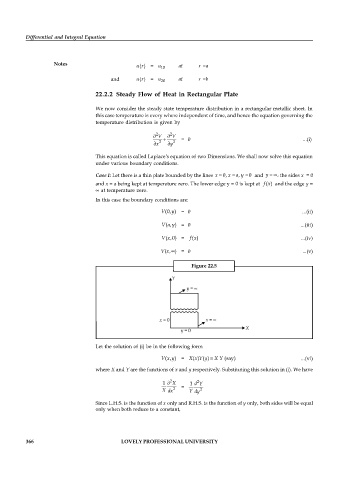
Case I: Let there is a thin plate bounded by the lines x 0, x , a y 0 and y , the sides x = 0
and x = a being kept at temperature zero. The lower edge y = 0 is kept at ( )f x and the edge y =
at temperature zero.
In this case the boundary conditions are:

y
V (0, ) = 0 ...(ii)
a
y
V ( , ) = 0 ...(iii)
V ( ,0) = f ( ) ...(iv)
x
x
x
V ( , ) = 0 ...(v)
Figure 22.5

Let the solution of (i) be in the following form
Y
y
x
y
V ( , ) = X ( ) ( ) X Y (say) ...(vi)
x
where X and Y are the functions of x and y respectively. Substituting this solution in (i). We have
2
1 2 X 1 d Y
2 = 2
X dx Y dy
Since L.H.S. is the function of x only and R.H.S. is the function of y only, both sides will be equal
only when both reduce to a constant,

366 LOVELY PROFESSIONAL UNIVERSITY