Page 376 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Unit 22: Solution of Laplace Differential Equation

Now using (xiv), we get Notes

n b n
x
V ( , ) = C n sin h sin x f ( )
b
x
n 1 a a
a
n b 2 n x
x
or C sin h = f ( ) sin dx
n
a a a
b
a
2 n x
or C n = n b f ( )sin dx ...(xvi)
x
a sinh 0 a
a
Hence (xv) with coefficient (xvi) in the solution of (i) satisfying the given boundary conditions.
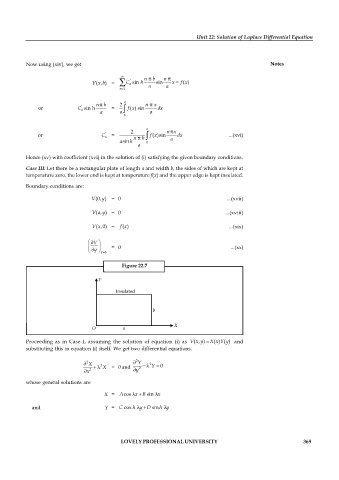
Case III: Let there be a rectangular plate of length a and width b, the sides of which are kept at
temperature zero, the lower end is kept at temperature f(x) and the upper edge is kept insulated.
Boundary conditions are:

V (0, ) = 0 ...(xvii)
y
y
V ( , ) = 0 ...(xviii)
a
V ( ,0) = f ( ) ...(xix)
x
x
V
= 0 ...(xx)
y
Y b
Figure 22.7

Proceeding as in Case I, assuming the solution of equation (i) as V ( , ) X ( ) ( ) and
Y
x
x
y
y
substituting this in equation (i) itself. We get two differential equations.
2
2 Y
X 2 X = 0 and 2 Y 0
x 2 y 2
whose general solutions are
X = A cos x B sin x

and Y = C cos h y D sinh y

LOVELY PROFESSIONAL UNIVERSITY 369