Page 296 - DCOM504_SECURITY_ANALYSIS_AND_PORTFOLIO_MANAGEMENT

Unit 11: Capital Market Theory

(f) The investors’ risk estimates are proportional to the variance of return they perceive Notes
for a security or portfolio.
(g) Investors base their investment decisions on two criteria i.e., expected return and
variance of return.
2. Efficient Frontier: Markowitz has formulised the risk return relationship and developed
the concept of efficient frontier. For selection of a portfolio, comparison between a
combination of portfolios is essential. As a rule, a portfolio is not efficient if there is
another portfolio with:
(a) a higher expected value of return and a lower standard deviation (risk)
(b) a higher expected value of return and the same standard deviations (risk).
(c) the same expected value but a lower standard deviation (risk).

Markowitz has defined the diversification as the process of combining assets that are less
than perfectly positively correlated in order to reduce portfolio risk without sacrificing
any portfolio returns. If an investor’s portfolio is not efficient he may:
(a) increase the expected value of return without increasing the risk.
(b) decrease the risk without decreasing the expected value of return, or
(c) obtain some combination of increase of expected return and decreased risk.

This is possible by switching to a portfolio on the efficient frontier.
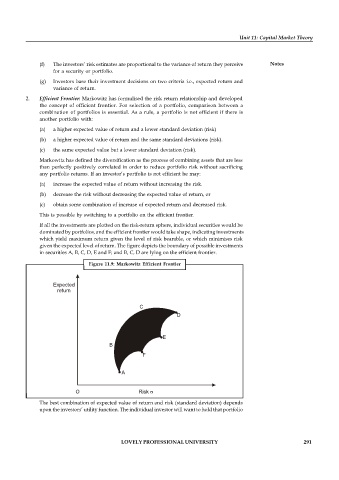
If all the investments are plotted on the risk-return sphere, individual securities would be
dominated by portfolios, and the efficient frontier would take shape, indicating investments
which yield maximum return given the level of risk bearable, or which minimises risk
given the expected level of return. The figure depicts the boundary of possible investments
in securities A, B, C, D, E and F; and B, C, D are lying on the efficient frontier.

Figure 11.9: Markowitz Efficient Frontier

Expected
return

C
D

E
B
F

A

O Risk

The best combination of expected value of return and risk (standard deviation) depends
upon the investors’ utility function. The individual investor will want to hold that portfolio

LOVELY PROFESSIONAL UNIVERSITY 291