Page 134 - DMTH202_BASIC_MATHEMATICS_II

Unit 10: Homogeneous Equations

Notes
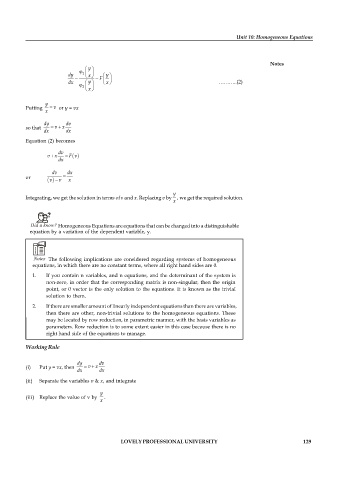
y
 
  
y
dy 1    
x
  F  
y
dx     ………..(2)
x
 2  
x
 
y
Putting  v or y = vx
x
dy dv
v
so that   x
dx dx
Equation (2) becomes
dv
v  x  F   v
dx
dv dx
or 
  v v x
y
Integrating, we get the solution in terms of v and x. Replacing v by , we get the required solution.
x

Did u know? Homogeneous Equations are equations that can be changed into a distinguishable
equation by a variation of the dependent variable, y.

Notes The following implications are considered regarding systems of homogeneous
equations, in which there are no constant terms, where all right hand sides are 0.
1. If you contain n variables, and n equations, and the determinant of the system is
non-zero, in order that the corresponding matrix is non-singular, then the origin
point, or 0 vector is the only solution to the equations. It is known as the trivial
solution to them.

2. If there are smaller amount of linearly independent equations than there are variables,
then there are other, non-trivial solutions to the homogeneous equations. These
may be located by row reduction, in parametric manner, with the basis variables as
parameters. Row reduction is to some extent easier in this case because there is no
right hand side of the equations to manage.

Working Rule

dy dv
v
(i) Put y = vx, then   x
dx dx
(ii) Separate the variables v & x, and integrate

y
(iii) Replace the value of v by .
x

LOVELY PROFESSIONAL UNIVERSITY 129