Page 95 - DMTH202_BASIC_MATHEMATICS_II

Basic Mathematics-II Sachin Kaushal, Lovely Professional University

Notes Unit 7: Definite Integral Applications

CONTENTS
Objectives
Introduction

7.1 Area under Simple Curve
7.2 Area within Two or more Curves
7.3 Summary
7.4 Keyword

7.5 Review Questions
7.6 Further Readings

Objectives

After studying this unit, you will be able to:
 Understand the application in finding the area under simple curve

 Discuss the application in finding the area within two curves

Introduction

Applications of the definite integral include finding the area under simple curve and finding the
area within two curves. In this unit, you will understand definite integral applications in detail
with explained examples.
7.1 Area under Simple Curve

We begin with the area under a simple curve, a straight line. A straight line, y = const, above a
distance in x is a rectangle and the area of a rectangle is the height multiplied by the width. For
some more anticipation appearance at the area under, or inside, a triangle created by the
coordinate axes and y = kx. These two areas, the rectangle and the triangle, include straight
2
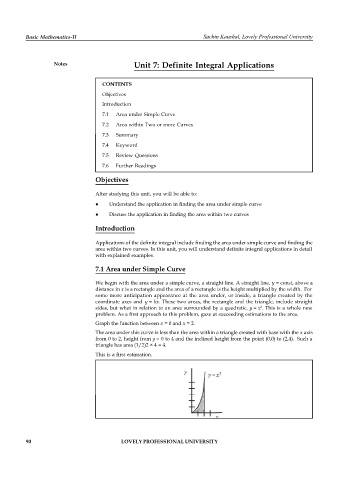
sides, but what in relation to an area surrounded by a quadratic, y = x . This is a whole new
problem. As a first approach to this problem, gaze at succeeding estimations to the area.
Graph the function between x = 0 and x = 2.
The area under this curve is less than the area within a triangle created with base with the x axis
from 0 to 2, height from y = 0 to 4 and the inclined height from the point (0,0) to (2,4). Such a
triangle has area (1/2)2 × 4 = 4.
This is a first estimation.

90 LOVELY PROFESSIONAL UNIVERSITY