Page 81 - DMGT402_MANAGEMENT_PRACTICES_AND_ORGANIZATIONAL_BEHAVIOUR

Management Practices and Organisational Behaviour

Notes 3.12 Decision Graphs

Probabilistic graphical models and decision graphs are powerful modeling tools for reasoning
and decision making under uncertainty.

Decision Tree Analysis

A decision tree is a decision support tool that uses a tree-like graph or model of decisions and
their possible consequences, including chance event outcomes, resource costs, and utility. A
decision tree is drawn only from left to right, and has only burst nodes (splitting paths) but no
sink nodes (converging paths). Therefore, if drawn manually, it can grow very big and become
hard to draw fully.

A decision tree is used as a visual and analytical tool, where the expected values (or expected
utility) of competing alternatives are calculated.
A decision tree consists of three types of nodes:

1. Decision nodes - commonly represented by squares
2. Chance nodes - represented by circles
3. End nodes - represented by triangles

Decision trees show the possible outcomes of different choices, taking into account probabilities,
costs and returns. They enable a manager to set out the consequences of choices, ensuring that he
has considered all possibilities and to assess the likelihood of each different possibility and to
assess the result of each possibility in terms of cost and profit.
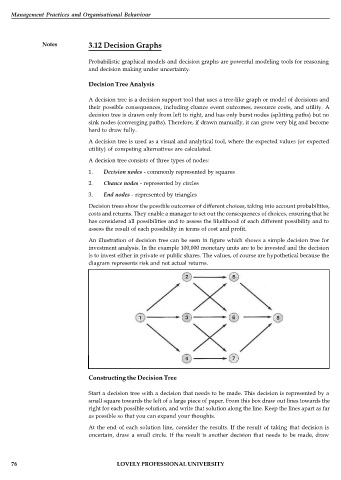
An illustration of decision tree can be seen in figure which shows a simple decision tree for
investment analysis. In the example 100,000 monetary units are to be invested and the decision
is to invest either in private or public shares. The values, of course are hypothetical because the
diagram represents risk and not actual returns.

Constructing the Decision Tree

Start a decision tree with a decision that needs to be made. This decision is represented by a
small square towards the left of a large piece of paper. From this box draw out lines towards the
right for each possible solution, and write that solution along the line. Keep the lines apart as far
as possible so that you can expand your thoughts.
At the end of each solution line, consider the results. If the result of taking that decision is
uncertain, draw a small circle. If the result is another decision that needs to be made, draw

76 LOVELY PROFESSIONAL UNIVERSITY