Page 136 - DLIS401_METHODOLOGY_OF_RESEARCH_AND_STATISTICAL_TECHNIQUES

Unit 9: Presentation of Data

Let us consider the score of 120 students of class X of a school in Mathematics, shown in Notes
Table 9.3.
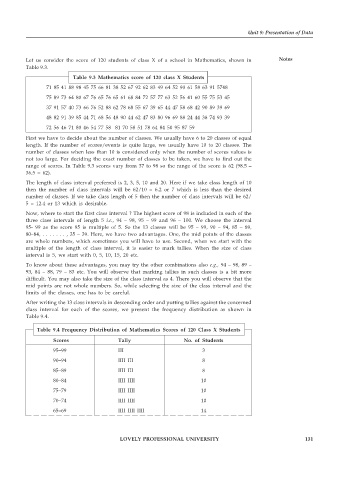
Table 9.3 Mathematics score of 120 class X Students
71 85 41 88 98 45 75 66 81 38 52 67 92 62 83 49 64 52 90 61 58 63 91 5748

75 89 73 64 80 67 76 65 76 65 61 68 84 72 57 77 63 52 56 41 60 55 75 53 45
37 91 57 40 73 66 76 52 88 62 78 68 55 67 39 65 44 47 58 68 42 90 89 39 69
48 82 91 39 85 44 71 68 56 48 90 44 62 47 83 80 96 69 88 24 44 38 74 93 39
72 56 46 71 80 46 54 77 58 81 70 58 51 78 64 84 50 95 87 59

First we have to decide about the number of classes. We usually have 6 to 20 classes of equal
length. If the number of scores/events is quite large, we usually have 10 to 20 classes. The
number of classes when less than 10 is considered only when the number of scores values is
not too large. For deciding the exact number of classes to be taken, we have to find out the
range of scores. In Table 9.3 scores vary from 37 to 98 so the range of the score is 62 (98.5 –
36.5 = 62).
The length of class interval preferred is 2, 3, 5, 10 and 20. Here if we take class length of 10
then the number of class intervals will be 62/10 = 6.2 or 7 which is less than the desired
rumber of classes. If we take class length of 5 then the number of class intervals will be 62/
5 = 12.4 or 13 which is desirable.
Now, where to start the first class interval ? The highest score of 98 is included in each of the
three class intervals of length 5 i.e., 94 – 98, 95 – 99 and 96 – 100. We choose the interval
95- 99 as the score 95 is multiple of 5. So the 13 classes will be 95 – 99, 90 – 94, 85 – 89,
80–84, . . . . . . . , 35 – 39. Here, we have two advantages. One, the mid points of the classes
are whole numbers, which sometimes you will have to use. Second, when we start with the
multiple of the length of class interval, it is easier to mark tallies. When the size of class
interval is 5, we start with 0, 5, 10, 15, 20 etc.
To know about these advantages, you may try the other combinations also e.g., 94 – 98, 89 –
93, 84 – 88, 79 – 83 etc. You will observe that marking tallies in such classes is a bit more
difficult. You may also take the size of the class interval as 4. There you will observe that the
mid points are not whole numbers. So, while selecting the size of the class interval and the
limits of the classes, one has to be careful.
After writing the 13 class intervals in descending order and putting tallies against the concerned
class interval for each of the scores, we present the frequency distribution as shown in
Table 9.4.

Table 9.4 Frequency Distribution of Mathematics Scores of 120 Class X Students
Scores Tally No. of Students
95–99 III 3
90–94 IIII III 8
85–89 IIII III 8

80–84 IIII IIII 10
75–79 IIII IIII 10
70–74 IIII IIII 10
65–69 IIII IIII IIII 14

LOVELY PROFESSIONAL UNIVERSITY 131