Page 412 - DECO401_MICROECONOMIC_THEORY_ENGLISH

Unit-29: Insurance Choice and Risk

29.2 Choice between Insurance and Gambling: Notes
Friedman–Savage Hypothesis

Some people are risk averse and they spend their life to review their insurance protection and involve in
gambling in casino. This is a paradox because it indicates that people can be risk averse and risk loving
at a time. In fact, there is no paradox because the behaviour insurance which can be bought depends
upon their nature and cost as well as the game of gambling.
If a person buys an insurance policy then he wants to get rid from risk. But when he buys a lottery ticket then
he gets small occasion of big profit. Thus, he takes risk. Some persons take in both insurance and gambling
and thus they take risk and protect too. Why? Answer has given by Friedman–Savage Hypothesis. It tells
that for income the marginal utility comes down on a level. It increases between a level of income and any
high level and again decreases for all income over that upper level. This is shown in Fig. 29.4 as total utility
curve TU where utility is shown on horizontal axis and income is on vertical axis.

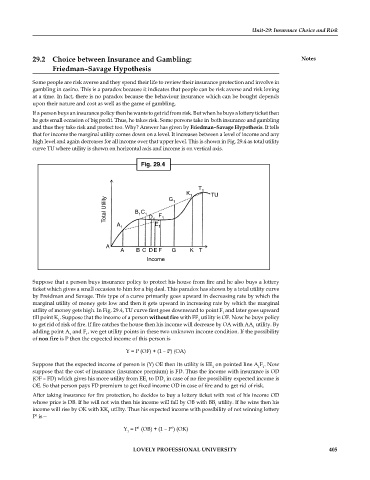
Fig. 29.4

T 1
K 1 TU
Total Utility B 1 C 1 D F
G
1

A 1 E 1
1 1

A
A B CDEF G K T
Income

Suppose that a person buys insurance policy to protect his house from fire and he also buys a lottery
ticket which gives a small occasion to him for a big deal. This paradox has shown by a total utility curve
by Freidman and Savage. This type of a curve primarily goes upward in decreasing rate by which the
marginal utility of money gets low and then it gets upward in increasing rate by which the marginal
utility of money gets high. In Fig. 29.4, TU curve first goes downward to point F and later goes upward
1
till point K . Suppose that the income of a person without fire with FF utility is OF. Now he buys policy
1
1
to get rid of risk of fire. If fire catches the house then his income will decrease by OA with AA utility. By
1
adding point A and F , we get utility points in these two unknown income condition. If the possibility
1
1
of non fire is P then the expected income of this person is
Y = P (OF) + (1 – P) (OA)
Suppose that the expected income of person is (Y) OE then its utility is EE on pointed line A F . Now
1
1 1
suppose that the cost of insurance (insurance premium) is FD. Thus the income with insurance is OD
(OF – FD) which gives his more utility from EE to DD in case of no fire possibility expected income is
1 1
OE. So that person pays FD premium to get fixed income OD in case of fire and to get rid of risk.
After taking insurance for fire protection, he decides to buy a lottery ticket with rest of his income OD
whose price is DB. If he will not win then his income will fall by OB with BB utility. If he wins then his
1
income will rise by OK with KK utility. Thus his expected income with possibility of not winning lottery
1
1
P is—
Y = P (OB) + (1 – P ) (OK)
1
1
1
LOVELY PROFESSIONAL UNIVERSITY 405