Page 177 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 11: Rank Correlation Method

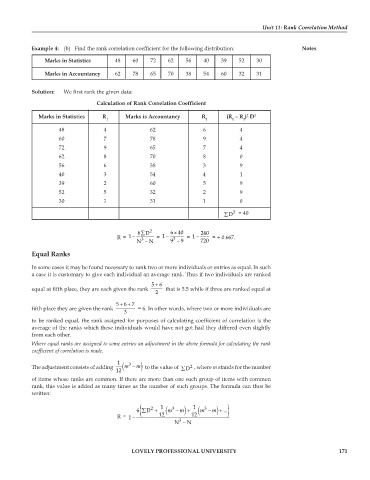
Example 4: (b) Find the rank correlation coefficient for the following distribution: Notes

Marks in Statistics 48 60 72 62 56 40 39 52 30
Marks in Accountancy 62 78 65 70 38 54 60 32 31

Solution: We first rank the given data:

Calculation of Rank Correlation Coefficient
Marks in Statistics R Marks is Accountancy R (R – R ) D 2
2
1 2 1 2
48 4 62 6 4
60 7 78 9 4
72 9 65 7 4
62 8 70 8 0
56 6 38 3 9
40 3 54 4 1
39 2 60 5 9
52 5 32 2 9
30 1 31 1 0
2
∑D = 40

×
∑
6D 2 640 240
R= −1 = 1 − 3 = −1 = + 0.667.
N 3 − N 9 − 9 720
Equal Ranks
In some cases it may be found necessary to rank two or more individuals or entries as equal. In such
a case it is customary to give each individual an average rank. Thus if two individuals are ranked
+
56
equal at fifth place, they are each given the rank that is 5.5 while if three are ranked equal at
2
++ 7
56
fifth place they are given the rank = 6. In other words, where two or more individuals are
3
to be ranked equal, the rank assigned for purposes of calculating coefficient of correlation is the
average of the ranks which these individuals would have not got had they differed even slightly
from each other.
Where equal ranks are assigned to some entries an adjustment in the above formula for calculating the rank
coefficient of correlation is made.
The adjustment consists of adding ( 1 3 − m to the value of 2 , where m stands for the number
) m
12 ∑D
of items whose ranks are common. If there are more than one such group of items with common
rank, this value is added as many times as the number of such groups. The formula can thus be
written:

∑ 6 2 +D 1 ( { 3 −m ) + 1 ( m 3 −m ) + m ... }
1
R= − 12 12
N 3 − N

LOVELY PROFESSIONAL UNIVERSITY 171