Page 179 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 11: Rank Correlation Method

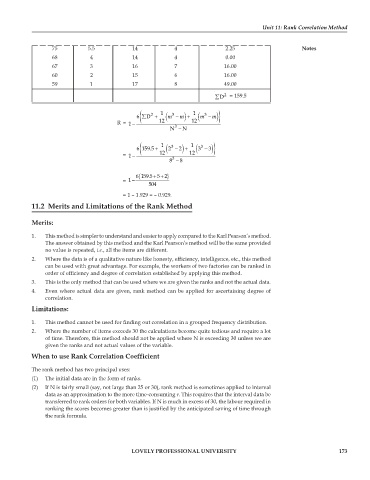
75 5.5 14 4 2.25 Notes
68 4 14 4 0.00
67 3 16 7 16.00
60 2 15 6 16.00
59 1 17 8 49.00
∑D = 159.5
2

∑ 6 2 +D 1 ( { 3 − ) m + 1 ( m 3 − m ) m }
R= − 12 12
1
N 3 − N
1 ( { 1 }
+ 6159.5 3 − 2 ) ( +2 3 − 3 ) 3
1
= − 12 12
8 3 − 8
{ ++ } 6 159.5 5 2
= 1−
504

= 1 – 1.929 = – 0.929.
11.2 Merits and Limitations of the Rank Method

Merits:

1. This method is simpler to understand and easier to apply compared to the Karl Pearson’s method.
The answer obtained by this method and the Karl Pearson’s method will be the same provided
no value is repeated, i.e., all the items are different.
2. Where the data is of a qualitative nature like honesty, efficiency, intelligence, etc., this method
can be used with great advantage. For example, the workers of two factories can be ranked in
order of efficiency and degree of correlation established by applying this method.
3. This is the only method that can be used where we are given the ranks and not the actual data.
4. Even where actual data are given, rank method can be applied for ascertaining degree of
correlation.
Limitations:

1. This method cannot be used for finding out correlation in a grouped frequency distribution.
2. Where the number of items exceeds 30 the calculations become quite tedious and require a lot
of time. Therefore, this method should not be applied where N is exceeding 30 unless we are
given the ranks and not actual values of the variable.

When to use Rank Correlation Coefficient

The rank method has two principal uses:
(1) The initial data are in the form of ranks.
(2) If N is fairly small (say, not large than 25 or 30), rank method is sometimes applied to interval
data as an approximation to the more time-consuming r. This requires that the interval data be
transferred to rank orders for both variables. If N is much in excess of 30, the labour required in
ranking the scores becomes greater than is justified by the anticipated saving of time through
the rank formula.

LOVELY PROFESSIONAL UNIVERSITY 173