Page 57 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 5: Application of Mean, Median and Mode

Notes
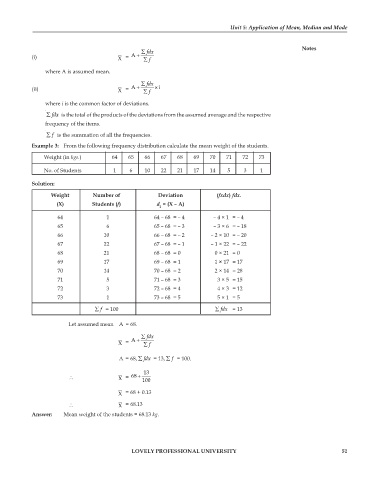
∑ fdx
(i) X = A + ∑ f

where A is assumed mean.

∑ fdx
(ii) X = A + ∑ f × i

where i is the common factor of deviations.
∑ fdx is the total of the products of the deviations from the assumed average and the respective
frequency of the items.
∑ f is the summation of all the frequencies.
Example 3: From the following frequency distribution calculate the mean weight of the students.

Weight (in kgs.) 64 65 66 67 68 69 70 71 72 73
No. of Students 1 6 10 22 21 17 14 5 3 1

Solution:

Weight Number of Deviation (fxdx) fdx.
(X) Students (f) d = (X – A)
2
64 1 64 – 68 = – 4 – 4 × 1 = – 4
65 6 65 – 68 = – 3 – 3 × 6 = – 18
66 10 66 – 68 = – 2 – 2 × 10 = – 20
67 22 67 – 68 = – 1 – 1 × 22 = – 22
68 21 68 – 68 = 0 0 × 21 = 0
69 17 69 – 68 = 1 1 × 17 = 17
70 14 70 – 68 = 2 2 × 14 = 28
71 5 71 – 68 = 3 3 × 5 = 15
72 3 72 – 68 = 4 4 × 3 = 12
73 1 73 – 68 = 5 5 × 1 = 5
∑ f = 100 ∑ fdx = 13

Let assumed mean A = 68.

∑ fdx
+
X = A ∑ f
A = 68, ∑ fdx = 13, ∑ f = 100.

13
∴ X = 68 + 100

X = 68 + 0.13

∴ X = 68.13
Answer: Mean weight of the students = 68.13 kg.

LOVELY PROFESSIONAL UNIVERSITY 51