Page 59 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 5: Application of Mean, Median and Mode

Solution: Notes

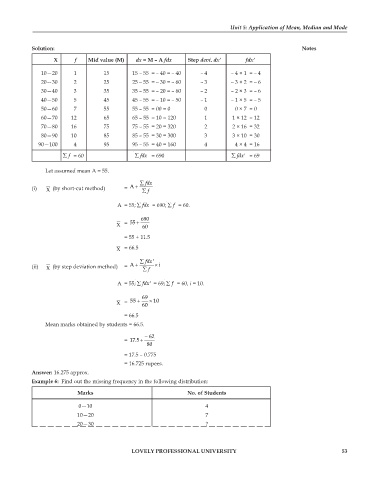
X f Mid value (M) dx = M – A fdx Step devi. dx’ fdx’
10—20 1 15 15 – 55 = – 40 = – 40 – 4 – 4 × 1 = – 4
20—30 2 25 25 – 55 = – 30 = – 60 – 3 – 3 × 2 = – 6
30—40 3 35 35 – 55 = – 20 = – 60 – 2 – 2 × 3 = – 6
40—50 5 45 45 – 55 = – 10 = – 50 – 1 – 1 × 5 = – 5
50—60 7 55 55 – 55 = 00 = 0 0 0 × 7 = 0
60—70 12 65 65 – 55 = 10 = 120 1 1 × 12 = 12
70—80 16 75 75 – 55 = 20 = 320 2 2 × 16 = 32
80—90 10 85 85 – 55 = 30 = 300 3 3 × 10 = 30
90—100 4 95 95 – 55 = 40 = 160 4 4 × 4 = 16
∑ f = 60 ∑ fdx = 690 ∑ fdx ' = 69

Let assumed mean A = 55.

∑ fdx
(i) X (by short-cut method) = A + ∑ f

A = 55; ∑ fdx = 690; ∑ f = 60.
690
X = 55 + 60
= 55 + 11.5

X = 66.5
∑ fdx '
(ii) X (by step deviation method) = A + ∑ f × i

'
A = 55; ∑ fdx = 69; ∑ f = 60, i = 10.
69
X = 55 + 60 × 10
= 66.5
Mean marks obtained by students = 66.5.

− 62
= 17.5 +
80
= 17.5 – 0.775
= 16.725 rupees.
Answer: 16.275 approx.
Example 6: Find out the missing frequency in the following distribution:
Marks No. of Students

0—10 4
10—20 7
20—30 ?

LOVELY PROFESSIONAL UNIVERSITY 53