Page 61 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 5: Application of Mean, Median and Mode

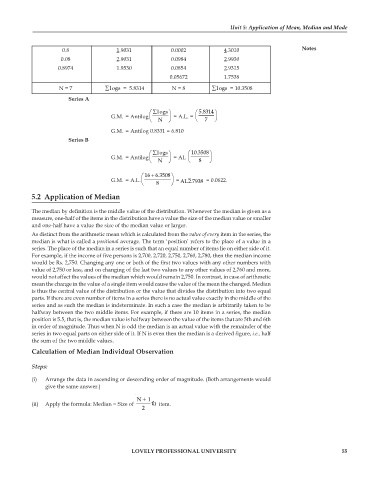
0.8 1.9031 0.0002 4.3010 Notes
0.08 2.9031 0.0984 2.9930
0.8974 1.9530 0.0854 2.9315
0.05672 1.7538

N = 7 ∑logs = 5.8314 N = 8 ∑logs = 10.3508
Series A
⎛ ∑logs ⎞ ⎛ ⎞ 5.8314
G.M. = Antilog ⎜ ⎟ = A.L. = ⎜ ⎟
⎝ N ⎠ ⎝ 7 ⎠
G.M. = Antilog 0.8331 = 6.810
Series B

⎛ ∑logs ⎞ ⎛ ⎞ 10.3508
G.M. = Antilog ⎜ ⎟ = AL ⎜ ⎟
⎝ N ⎠ ⎝ 8 ⎠

⎛ + 6.3508 ⎞ 16
G.M. = A.L. ⎜ ⎟ = AL2.7938 = 0.0622.
⎝ 8 ⎠
5.2 Application of Median

The median by definition is the middle value of the distribution. Whenever the median is given as a
measure, one-half of the items in the distribution have a value the size of the median value or smaller
and one-half have a value the size of the median value or larger.
As distinct from the arithmetic mean which is calculated from the value of every item in the series, the
median is what is called a positional average. The term ‘position’ refers to the place of a value in a
series. The place of the median in a series is such that an equal number of items lie on either side of it.
For example, if the income of five persons is 2,700, 2,720, 2,750, 2,760, 2,780, then the median income
would be Rs. 2,750. Changing any one or both of the first two values with any other numbers with
value of 2,750 or less, and on changing of the last two values to any other values of 2,760 and more,
would not affect the values of the median which would remain 2,750. In contrast, in case of arithmetic
mean the change in the value of a single item would cause the value of the mean the changed. Median
is thus the central value of the distribution or the value that divides the distribution into two equal
parts. If there are even number of items in a series there is no actual value exactly in the middle of the
series and as such the median is indeterminate. In such a case the median is arbitrarily taken to be
halfway between the two middle items. For example, if there are 10 items in a series, the median
position is 5.5, that is, the median value is halfway between the value of the items that are 5th and 6th
in order of magnitude. Thus when N is odd the median is an actual value with the remainder of the
series in two equal parts on either side of it. If N is even then the median is a derived figure, i.e., half
the sum of the two middle values.
Calculation of Median Individual Observation

Steps:

(i) Arrange the data in ascending or descending order of magnitude. (Both arrangements would
give the same answer.)

N + 1
(ii) Apply the formula: Median = Size of th item.
2

LOVELY PROFESSIONAL UNIVERSITY 55