Page 58 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Statistical Methods in Economics

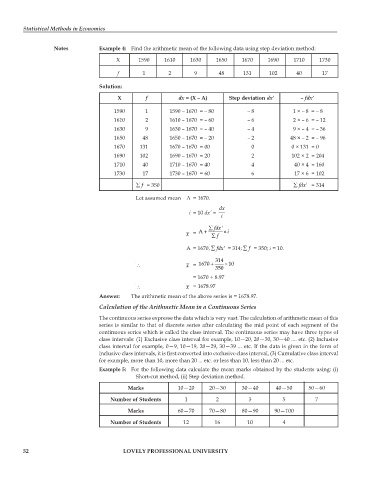
Notes Example 4: Find the arithmetic mean of the following data using step deviation method:

X 1590 1610 1630 1650 1670 1690 1710 1730
f 1 2 9 48 131 102 40 17

Solution:

X f dx = (X – A) Step deviation dx’ – fdx’
1590 1 1590 – 1670 = – 80 – 8 1 × – 8 = – 8
1610 2 1610 – 1670 = – 60 – 6 2 × – 6 = – 12
1630 9 1630 – 1670 = – 40 – 4 9 × – 4 = – 36
1650 48 1650 – 1670 = – 20 – 2 48 × – 2 = – 96
1670 131 1670 – 1670 = 00 0 0 × 131 = 0
1690 102 1690 – 1670 = 20 2 102 × 2 = 204
1710 40 1710 – 1670 = 40 4 40 × 4 = 160
1730 17 1730 – 1670 = 60 6 17 × 6 = 102

∑ f = 350 ∑ fdx ' = 314
Let assumed mean A = 1670.
dx
i = 10 dx’ =
i
∑ fdx '
x = A + ∑ f × i
'
A = 1670, ∑ fdx = 314; ∑ f = 350; i = 10.
314
∴ x = 1670 + 350 × 10
= 1670 + 8.97
∴ x = 1678.97
Answer: The arithmetic mean of the above series is = 1678.97.
Calculation of the Arithmetic Mean in a Continuous Series

The continuous series expresse the data which is very vast. The calculation of arithmetic mean of this
series is similar to that of discrete series after calculating the mid point of each segment of the
continuous series which is called the class interval. The continuous series may have three types of
class intervals: (1) Exclusive class interval for example, 10—20, 20—30, 30—40 .... etc. (2) Inclusive
class interval for example, 0—9, 10—19, 20—29, 30—39 ... etc. If the data is given in the form of
inclusive class intervals, it is first converted into exclusive class interval, (3) Cumulative class interval
for example, more than 10, more than 20 ... etc. or less than 10, less than 20 ... etc.
Example 5: For the following data calculate the mean marks obtained by the students using: (i)
Short-cut method, (ii) Step deviation method.
Marks 10—20 20—30 30—40 40—50 50—60

Number of Students 1 2 3 5 7
Marks 60—70 70—80 80—90 90—100
Number of Students 12 16 10 4

52 LOVELY PROFESSIONAL UNIVERSITY