Page 207 - DMTH401_REAL ANALYSIS

Unit 15: Uniform Convergence of Functions

Notes
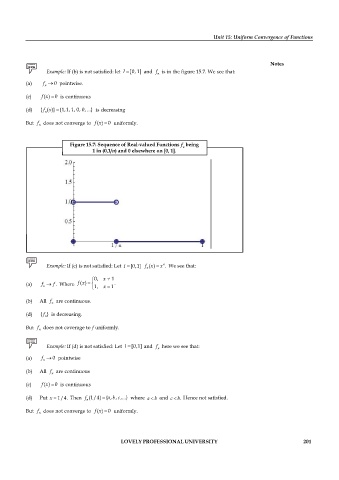
Example: If (b) is not satisfied: let I = [0, 1] and f is in the figure 15.7. We see that:
n
0
(a) f  pointwise.
n
x
=
(c) f ( ) 0 is continuous
(d) { ( )} {1,1, 1, 0, 0, ...} is decreasing
x
f
=
n
But f does not converge to ( ) 0f x = uniformly.
n
Figure 15.7: Sequence of Real-valued Functions f being
n
1 in (0,1/n) and 0 elsewhere on [0, 1].

n
x
Example: If (c) is not satisfied: Let I = [0,1] f ( ) x We see that:
.
=
n
ì 0, x ¹ 1
x
(a) f  . f Where f ( ) = í .
n
î 1, x = 1
(b) All f are continuous.
n
f
(d) { } is decreasing.
n
But f does not coverage to f uniformly.
n
Example: If (d) is not satisfied: Let I = [0,1] and f here we see that:
n
(a) f  0 pointwise
n
(b) All f are continuous
n
x
(c) f ( ) 0 is continuous
=
a
c
b
=
(d) Put x = 1/4. Then f n (1/4) { , , ,...} where a b< and c < . b Hence not satisfied.
But f does not converge to ( ) 0f x = uniformly.
n

LOVELY PROFESSIONAL UNIVERSITY 201