Page 204 - DMTH401_REAL ANALYSIS

Real Analysis

Notes
f ( ) - f ( ) + f ( ) - f ( ) + f ( ) - f ( ) £ e /3 + e /3 + e /3 = e .
y
y
x
y
x
x
N N N N
Remark: This theorem also work for f defined on any I   . Fix x Î . I This can be either
n
isolated point in I or the limit point of I. In both cases we use exactly the same argument as for
b
[ , ] case.
a
We should compare uniform with pointwise convergence:
 For pointwise convergence we could first fix a value for x and then choose N. Consequently,
N depends on both and x.
 For uniform convergence f (x) must be uniformly close to f(x) for all x in the domain. Thus
n
N only depends on but not on x.
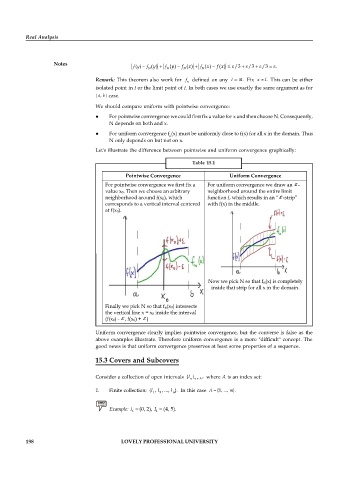
Let's illustrate the difference between pointwise and uniform convergence graphically:
Table 15.1
Pointwise Convergence Uniform Convergence
For pointwise convergence we first fix a For uniform convergence we draw an -
value x 0. Then we choose an arbitrary neighborhood around the entire limit
neighborhood around f(x 0), which function f, which results in an “ -strip”
corresponds to a vertical interval centered with f(x) in the middle.
at f(x 0).

Now we pick N so that f n(x) is completely
inside that strip for all x in the domain.

Finally we pick N so that f n(x 0) intersects
the vertical line x = x 0 inside the interval
(f(x 0) - , f(x 0) + )

Uniform convergence clearly implies pointwise convergence, but the converse is false as the
above examples illustrate. Therefore uniform convergence is a more "difficult" concept. The
good news is that uniform convergence preserves at least some properties of a sequence.
15.3 Covers and Subcovers

Consider a collection of open intervals { }I a a Î A , where A is an index set:

1. Finite collection: { ,I I , ..., I }. In this case A = {1, ..., m }.
1 2 m

I = (0, 2), I = (4, 5).
Example: 1
2

198 LOVELY PROFESSIONAL UNIVERSITY