Page 240 - DMTH401_REAL ANALYSIS

Real Analysis

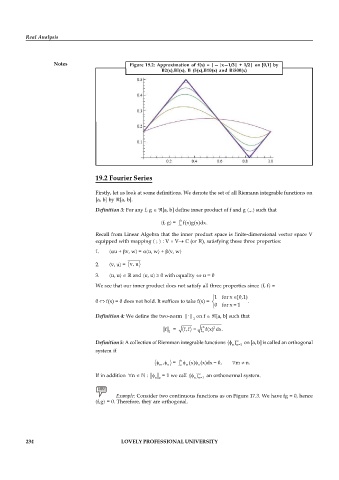
Notes Figure 19.2: Approximation of f(x) = |—|x—1/3| + 1/2| on [0,1] by
B2(x),BI(x), B (5(x),B10(x) and Bi500(x)

19.2 Fourier Series

Firstly, let us look at some definitions. We denote the set of all Riemann integrable functions on
[a, b] by [a, b].

Definition 3: For any f, g Î [a, b] define inner product of f and g .,. such that
b
f, g = ò f(x)g(x)dx.
a
Recall from Linear Algebra that the inner product space is finite-dimensional vector space V
equipped with mapping ·,·: V  V  (or ), satisfying these three properties:

1. u + v, w = u, w + v, w

2. v, u = v, u
3. u, u Î  and u, u  0 with equality  u = 0
We see that our inner product does not satisfy all three properties since f, f =

Î
ì 1 for x [0,1)
0 f(x) = 0 does not hold. It suffices to take f(x) = í .
î 0 for x = 1
Definition 4: We define the two-norm ||· || on f Î[a, b] such that
2
2
f = f,f = a ò b f(x) dx.
2
Definition 5: A collection of Riemman integrable functions {f } ¥ on [a, b] is called an orthogonal
=
n n 1
system if
b
f
f m ,f n = ò a f m (x) (x)dx = 0, " m ¹ n.
n
If in addition n" Î : f = 1 we call {f } ¥ an orthonormal system.
=
n 2 n n 1
Example: Consider two continuous functions as on Figure 17.3. We have fg = 0, hence
f,g = 0. Therefore, they are orthogonal.

234 LOVELY PROFESSIONAL UNIVERSITY