Page 108 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Unit 10: Residues and Singularities

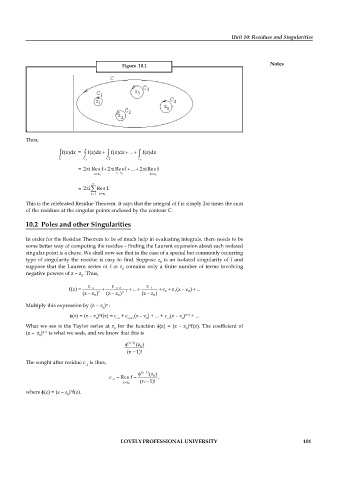
Figure 10.1 Notes

Then,

 f(z)dz =  f(z)dz   f(z)dz ...   f(z)dz

C C 1 C2 C n
= 2 i Res f 2 iResf ... 2 iRes f     
z 1 z z 2 z z n z
n
= 2 i  Res f.
k 1 z k z

This is the celebrated Residue Theorem. It says that the integral of f is simply 2i times the sum
of the residues at the singular points enclosed by the contour C.

10.2 Poles and other Singularities

In order for the Residue Theorem to be of much help in evaluating integrals, there needs to be
some better way of computing the residue—finding the Laurent expansion about each isolated
singular point is a chore. We shall now see that in the case of a special but commonly occurring
type of singularity the residue is easy to find. Suppose z is an isolated singularity of f and
0
suppose that the Laurent series of f at z contains only a finite number of terms involving
0
negative powers of z – z . Thus,
0
c c c


f(z)   n   n 1  ...   1  c  c (z z ) ...

(z z ) n (z z ) n 1 (z z ) 0 1 0




0
0
0
Multiply this expression by (z – z ) :
n
0
(z) = (z – z ) f(z) = c + c –n+1 (z – z ) + ... + c (z – z ) + ...
n–1
n
0
0
–1
0
–n
What we see is the Taylor series at z for the function (z) = (z – z ) f(z). The coefficient of
n
0
0
(z – z ) is what we seek, and we know that this is
n–1
0

 (n 1) (z )
0
(n 1)!

The sought after residue c is thus,
–1

 (n 1) (z )
c  Res f  0 ,
 1 (n 1)!
z 0 z 
where (z) = (z – z ) f(z).
n
0
LOVELY PROFESSIONAL UNIVERSITY 101