Page 111 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

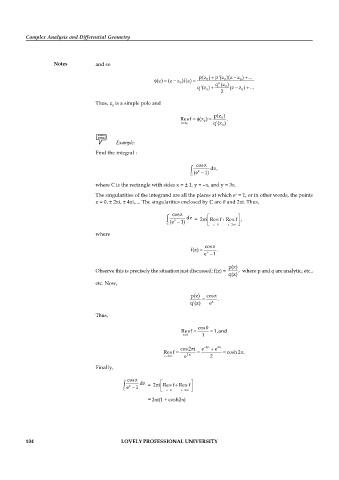
Notes and so

p(z ) p'(z )(z z ) ...



0

 (z) (z z )f(z)  0 q (z ) 0

0
n
q'(z )  2 0 (z z ) ...


0
0
Thus, z is a simple pole and
0
p(z )
Resf   (z )  0 .
0
z 0 z q'(z )
0
Example:
Find the integral :
cosz
 z 1) dz,
C (e 
where C is the rectangle with sides x = ± 1, y = –, and y = 3.
The singularities of the integrand are all the places at which e = 1, or in other words, the points
z
z = 0, ± 2i, ± 4i,.... The singularities enclosed by C are 0 and 2i. Thus,
cosz


 z 1) dz = 2 i Res f Res f ,


C (e    z 0 z 2 i  



where
cosz
f(z)  .
z
e  1
p(z)
Observe this is precisely the situation just discussed: f(z) = , where p and q are analytic, etc.,
q(z)
etc. Now,
p(z) cosz .
q'(z)  e z
Thus,

cos0
Resf   1,and
z 0 1

cos2 i e  2p  e 2

Resf    cosh 2 .


z 2 i  e 2 i  2
Finally,
cosz dz  
z 



e  1 = 2 i Res f Res f 
C  z 0 z 2 i 



= 2i(1 + cosh2)

104 LOVELY PROFESSIONAL UNIVERSITY