Page 260 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Unit 21: New Spherical Indicatrices and their Characterizations

In order to determine the Bishop frame of the curve  = (s), let us form Notes

s b bs
(s) = 2  ds  2 .
0 c c
Since, we can write the transformation matrix

 
 1 0 0 

 T    bs bs  T 

  cos 2 sin 2  
N 
    0 c c    M 1  ,
  bs bs  M 
B
    sin cos   2 
2
0  c 2 c 
 
 
by the method of Cramer, one can obtain the Bishop trihedra as follows:
The tangent:
1 s s
T  ( asin ,acos ,b) (13)

c c c
The M :
1
s bs b s bs b s bs s bs a bs
M = ( cos cos c c 2  c sin sin c 2 , cos sin c 2  sin cos c 2 , c sin c 2 )
c
c
c
c
1
The M :
2
b s bs s bs b s bs s bs a bs
M = ( sin cos c 2  cos sin c 2 , c cos cos c 2  sin sin c 2 , cos c 2 )
c
c
c
c
c
c
2
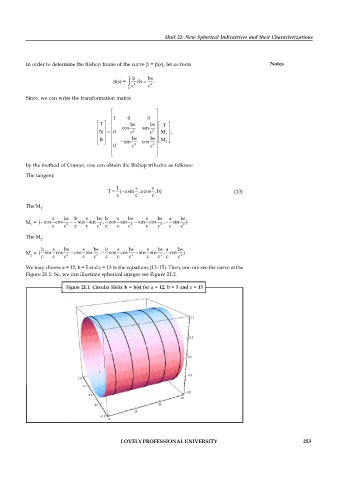
We may choose a = 12, b = 5 and c = 13 in the equations (12–15). Then, one can see the curve at the
Figure 21.1. So, we can illustrate spherical images see Figure 21.2.
Figure 21.1: Circular Helix b = b(s) for a = 12; b = 5 and c = 13

LOVELY PROFESSIONAL UNIVERSITY 253