Page 259 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

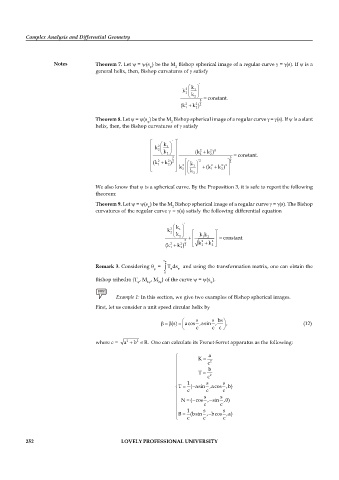
Notes Theorem 7. Let  = (s ) be the M Bishop spherical image of a regular curve  = (s). If  is a
2

general helix, then, Bishop curvatures of  satisfy
'

k 2 k  
1
2 
 k 2  constant.
3 
2
(k  k )
2 2
1
2
Theorem 8. Let  = (s ) be the M Bishop spherical image of a regular curve  = (s). If  is a slant
2

helix, then, the Bishop curvatures of  satisfy
 2 k  '  '

 k 2  1  
2
2 4
  k 2   (k  k )  constant.
1
2
 3  3
2
2 2
 (k  k )    k  '2 2 
1
2
2
3
2 3
    k  1   (k  k ) 
2 
2
1
 k 2   

We also know that  is a spherical curve. By the Proposition 3, it is safe to report the following
theorem:
Theorem 9. Let  = (s ) be the M Bishop spherical image of a regular curve  = (s). The Bishop
2

curvatures of the regular curve  = (s) satisfy the following differential equation
'

k 2 k   '
1
2 
 k 2     k k 2    constant.
1
3 2 2
2
2 
2 2
1
(k  k )   k  k 
2
1
s 
Remark 3. Considering  =  T ds and using the transformation matrix, one can obtain the
  
0
Bishop trihedra {T , M , M } of the curve  = (s ).
 1 2 
Example 1: In this section, we give two examples of Bishop spherical images.
First, let us consider a unit speed circular helix by
s
s bs 
   (s)    acos ,asin ,  , (12)
 c c c 
2
2
where c = a  b  R. One can calculate its Frenet-Serret apparatus as the following:
 a
 K  c 2

 T  b
 c 2

 1 s s

 T  ( asin ,acos ,b)
 c c c
 s s


 N  ( cos , sin ,0)
c
c

s
s
 1 (bsin , bcos ,a)
 B  c c  c

252 LOVELY PROFESSIONAL UNIVERSITY