Page 144 - DMTH404_STATISTICS

Statistics

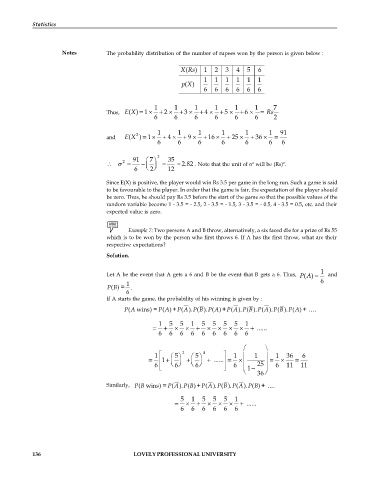
Notes The probability distribution of the number of rupees won by the person is given below :

X (Rs ) 1 2 3 4 5 6
1 1 1 1 1 1
p ( )
X
6 6 6 6 6 6

1 1 1 1 1 1 7
X
Thus, E ( ) 1´ + 2 ´ + 3 ´ + 4´ + 5´ + 6´ = Rs
=
6 6 6 6 6 6 2
1 1 1 1 1 1 91
2
=
and ( E X ) 1´ + 4 ´ + 9 ´ + 16 ´ + 25 ´ + 36 ´ =
6 6 6 6 6 6 6
2
7
91 æ ö 35
2
\  = - ç ÷ = = 2.82 . Note that the unit of  will be (Rs) .
2
2
6 è 2 ø 12
Since E(X) is positive, the player would win Rs 3.5 per game in the long run. Such a game is said
to be favourable to the player. In order that the game is fair, the expectation of the player should
be zero. Thus, he should pay Rs 3.5 before the start of the game so that the possible values of the
random variable become 1 - 3.5 = - 2.5, 2 - 3.5 = - 1.5, 3 - 3.5 = - 0.5, 4 - 3.5 = 0.5, etc. and their
expected value is zero.

Example 7: Two persons A and B throw, alternatively, a six faced die for a prize of Rs 55
which is to be won by the person who first throws 6. If A has the first throw, what are their
respective expectations?
Solution.

1
Let A be the event that A gets a 6 and B be the event that B gets a 6. Thus, P ( ) = and
A
1 6
P(B) = .
6
If A starts the game, the probability of his winning is given by :
P(A wins) = P(A)+P(A).P(B).P(A)+P(A).P(B).P(A).P(B).P(A)+ ....

1 5 5 1 5 5 5 5 1
= + ´ ´ + ´ ´ ´ ´ + ......
6 6 6 6 6 6 6 6 6

æ ö
1 é æ 5ö 2 æ 5ö 4 ù 1 ç 1 ÷ 1 36 6
= ê 1+ ç ÷ + ç ÷ + ...... = ´ ç ÷ = ´ =
ú
6 ê ë è 6 ø è 6 ø ú û 6 25 6 11 11
ç 1- ÷
è 36 ø
Similarly, P(B wins) = P(A).P(B)+P(A).P(B).P(A).P(B)+ ....

5 1 5 5 5 1
= ´ + ´ ´ ´ + ......
6 6 6 6 6 6

136 LOVELY PROFESSIONAL UNIVERSITY