Page 148 - DMTH404_STATISTICS

Statistics

Notes This gives us a conditional probability distribution of X given that Y = Y . This distribution can
1
be written in a tabular form as shown below :

X X X ... ... X Total Probability
1 2 m
p p p
Probability 11 21 ... ... m 1 1
P 1 P 1 P 1
The conditional distribution of X given some other value of Y can be constructed in a similar
way. Further, we can construct the conditional distributions of Y for various given values of X.

Remarks:
It can be shown that if the conditional distribution of a random variable is same as its marginal
distribution, the two random variables are independent. Thus, if for the conditional distribution
p
of X given Y we have 1 i = P for " i, then X and Y are independent. It should be noted here that
1 i
P 1
if one conditional distribution satisfies the condition of independence of the random variables,
then all the conditional distributions would also satisfy this condition.

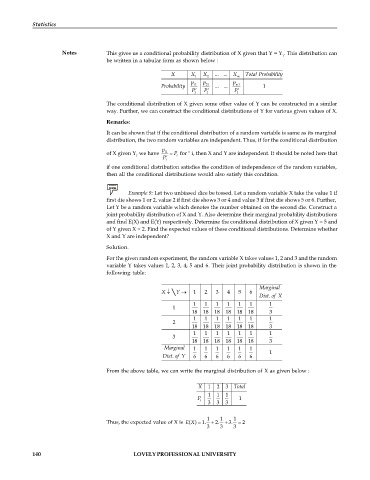
Example 9: Let two unbiased dice be tossed. Let a random variable X take the value 1 if
first die shows 1 or 2, value 2 if first die shows 3 or 4 and value 3 if first die shows 5 or 6. Further,
Let Y be a random variable which denotes the number obtained on the second die. Construct a
joint probability distribution of X and Y. Also determine their marginal probability distributions
and find E(X) and E(Y) respectively. Determine the conditional distribution of X given Y = 5 and
of Y given X = 2. Find the expected values of these conditional distributions. Determine whether
X and Y are independent?
Solution.

For the given random experiment, the random variable X takes values 1, 2 and 3 and the random
variable Y takes values 1, 2, 3, 4, 5 and 6. Their joint probability distribution is shown in the
following table:

Marginal
X ¯ \ Y ® 1 2 3 4 5 6 Dist . X
of
1 1 1 1 1 1 1
1
18 18 18 18 18 18 3
1 1 1 1 1 1 1
2
18 18 18 18 18 18 3
1 1 1 1 1 1 1
3
18 18 18 18 18 18 3
Marginal 1 1 1 1 1 1
1
Dist . Y 6 6 6 6 6 6
of
From the above table, we can write the marginal distribution of X as given below :

X 1 2 3 Total
1 1 1
P i 1
3 3 3

1 1 1
Thus, the expected value of X is ( ) 1.E X = + 2. + 3. = 2
3 3 3

140 LOVELY PROFESSIONAL UNIVERSITY