Page 214 - DMTH404_STATISTICS

Statistics

Notes 5. Since median = m, the ordinate at X = m divides the area under the normal curve into two
equal parts, i.e.,

m ¥
-¥ ò p X m ò p X 0.5
( )dX =
( )dX =
6. The value of p(X) is always non-negative for all values of X, i.e., the whole curve lies above
X axis.
7. The points of inflexion (the point at which curvature changes) of the curve are at X = m ±
 .
8. The quartiles are equidistant from median, i.e., M - Q = Q - M , by virtue of symmetry.
d 1 3 d
Also Q = m - 0.6745  , Q = m + 0.6745  , quartile deviation = 0.6745 and mean deviation
1 3
= 0.8s, approximately.
9. Since the distribution is symmetrical, all odd ordered central moments are zero.
10. The successive even ordered central moments are related according to the following
recurrence formula
m = (2n - 1)  m for n = 1, 2, 3, ......
2
2n 2n - 2
11. The value of moment coefficient of skewness b is zero.
1
m 3 4
12. The coefficient of kurtosis b = 4 = = 3.
2 2 4
m 
2
Note that the above expression makes use of property 10.
13. Additive or reproductive property
If X , X , ...... X are n independent normal variates with means m m, ,  m and variances
1 2 n 1 2 n
2
2
,
 2 1 ,   , respectively, then their linear combination a X + a X + ...... + a X is also
2
n
n n 1 1 2 2 n n
2
2
a normal variate with mean å a m and variance å a  .
i i i i
i= 1 i= 1
In particular, if a = a = ...... = a = 1, we have å X is a normal variate with mean å m and
1 2 n i i
2
variance å  . Thus the sum of independent normal variates is also a normal variate.
i
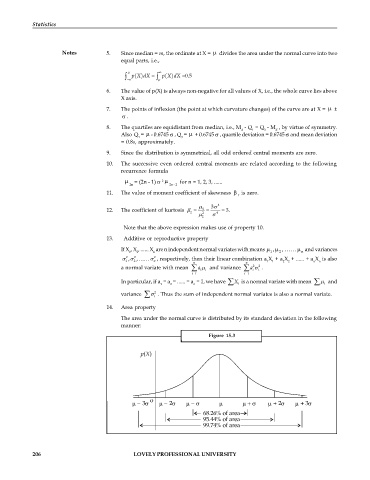
14. Area property
The area under the normal curve is distributed by its standard deviation in the following
manner:
Figure 15.3

206 LOVELY PROFESSIONAL UNIVERSITY