Page 217 - DMTH404_STATISTICS

Unit 15: Exponential Distribution and Normal Distribution

Notes

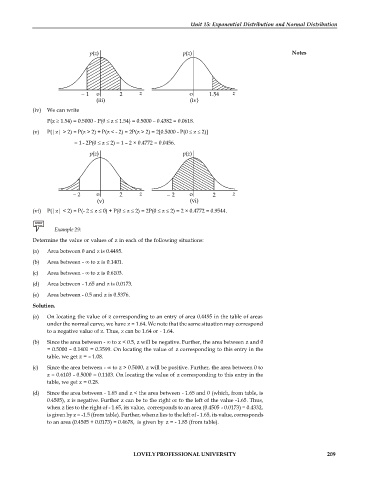
(iv) We can write
P(z  1.54) = 0.5000 - P(0 £ z £ 1.54) = 0.5000 – 0.4382 = 0.0618.

(v) P(|z| > 2) = P(z > 2) + P(z < - 2) = 2P(z > 2) = 2[0.5000 - P(0 £ z £ 2)]
= 1 - 2P(0 £ z £ 2) = 1 – 2 × 0.4772 = 0.0456.

(vi) P(|z| < 2) = P(- 2 £ z £ 0) + P(0 £ z £ 2) = 2P(0 £ z £ 2) = 2 × 0.4772 = 0.9544.

Example 29:
Determine the value or values of z in each of the following situations:
(a) Area between 0 and z is 0.4495.

(b) Area between - ¥ to z is 0.1401.
(c) Area between - ¥ to z is 0.6103.
(d) Area between - 1.65 and z is 0.0173.
(e) Area between - 0.5 and z is 0.5376.

Solution.
(a) On locating the value of z corresponding to an entry of area 0.4495 in the table of areas
under the normal curve, we have z = 1.64. We note that the same situation may correspond
to a negative value of z. Thus, z can be 1.64 or - 1.64.
(b) Since the area between - ¥ to z < 0.5, z will be negative. Further, the area between z and 0
= 0.5000 – 0.1401 = 0.3599. On locating the value of z corresponding to this entry in the
table, we get z = – 1.08.
(c) Since the area between - ¥ to z > 0.5000, z will be positive. Further, the area between 0 to
z = 0.6103 - 0.5000 = 0.1103. On locating the value of z corresponding to this entry in the
table, we get z = 0.28.

(d) Since the area between - 1.65 and z < the area between - 1.65 and 0 (which, from table, is
0.4505), z is negative. Further z can be to the right or to the left of the value -1.65. Thus,
when z lies to the right of - 1.65, its value, corresponds to an area (0.4505 - 0.0173) = 0.4332,
is given by z = -1.5 (from table). Further, when z lies to the left of - 1.65, its value, corresponds
to an area (0.4505 + 0.0173) = 0.4678, is given by z = - 1.85 (from table).

LOVELY PROFESSIONAL UNIVERSITY 209