Page 303 - DMTH404_STATISTICS

Unit 22: Correlation

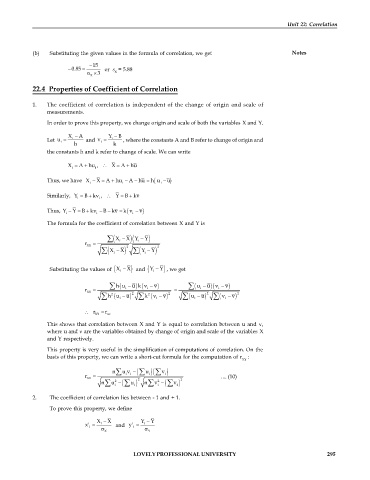
(b) Substituting the given values in the formula of correlation, we get Notes

 15
 0.85  or s = 5.88
  3 X
X
22.4 Properties of Coefficient of Correlation

1. The coefficient of correlation is independent of the change of origin and scale of
measurements.
In order to prove this property, we change origin and scale of both the variables X and Y.

X  A Y  B
Let u  i and v  i , where the constants A and B refer to change of origin and
i
i
h k
the constants h and k refer to change of scale. We can write


X  A hu ,  X  A hu
i
i
Thus, we have X  X  A  hu  A hu  h u  u 

i
i
i

Similarly, Y  B kv ,  Y  B kv

i
i
Thus, Y  Y  B kv  B kv  k v  v 


i
i
i
The formula for the coefficient of correlation between X and Y is
  X  X  Y  Y 
r  i i
XY 2 2
  X  X    Y  Y 
i
i
Substituting the values of  X  X  and  Y  Y  , we get
i
i
 h u  u k  v  u  u v   v
 v 
r  i i  i i
XY 2 2 2 2 2 2
 h u  u   k v   v  u  u   v   v
i
i
i
i
r  r
 XY uv

This shows that correlation between X and Y is equal to correlation between u and v,
where u and v are the variables obtained by change of origin and scale of the variables X
and Y respectively.
This property is very useful in the simplification of computations of correlation. On the
basis of this property, we can write a short-cut formula for the computation of r :
XY
n  u v   u i  v i 
i
i
r  .... (10)
XY 2 2
2
2
n  u   u i  n  v   v i 
i
i
2. The coefficient of correlation lies between - 1 and + 1.
To prove this property, we define
X  X Y  Y
x'  i and y'  i
i
i
 X  Y
LOVELY PROFESSIONAL UNIVERSITY 295