Page 306 - DMTH404_STATISTICS

Statistics

Notes (v) Sum of the product of deviations of X and Y values = 32

(vi) No. of pairs of observations = 10
(b) Given the following, calculate the coefficient of correlation :
(i) Sum of squares of deviations of X values from mean = 136

(ii) Sum of squares of deviations of Y values from mean = 138
(iii) Sum of products of deviations of X and Y values from their means = 122.
Solution.
(a) Let u = X - A and v = Y - B be the deviations of X and Y values. We are given Su = 5, Sv =
i i i i i i
4, Su = 40, Sv = 50, Su v = 32 and n = 10.
2
2
i i i i
Substituting these values in formula (10), we get


10 32 5 4

r   0.704
XY 2 2
10 40 5 10 50 4




122
(b) Using formula (3) for correlation, we get r   0.89
136 138
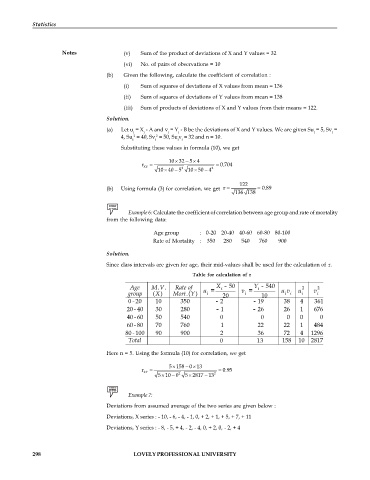
Example 6: Calculate the coefficient of correlation between age group and rate of mortality
from the following data:
Age group : 0-20 20-40 40-60 60-80 80-100
Rate of Mortality : 350 280 540 760 900
Solution.
Since class intervals are given for age, their mid-values shall be used for the calculation of r.
Table for calculation of r

Age M.V. Rate of X - 50 Y - 540 2 2
i
i
group (X) Mort.(Y) u = 20 v = 10 u v u i v i
i
i
i i
0 - 20 10 350 - 2 - 19 38 4 361
20 - 40 30 280 - 1 - 26 26 1 676
40 - 60 50 540 0 0 0 0 0
60 - 80 70 760 1 22 22 1 484
80 -100 90 900 2 36 72 4 1296
Total 0 0 13 13 158 10 2817
Here n = 5. Using the formula (10) for correlation, we get



5 158 0 13

r   0.95
XY 2 2
5 10 0 5 2817 13




Example 7:
Deviations from assumed average of the two series are given below :
Deviations, X series : - 10, - 6, - 4, - 1, 0, + 2, + 1, + 5, + 7, + 11
Deviations, Y series : - 8, - 5, + 4, - 2, - 4, 0, + 2, 0, - 2, + 4

298 LOVELY PROFESSIONAL UNIVERSITY