Page 311 - DMTH404_STATISTICS

Unit 22: Correlation

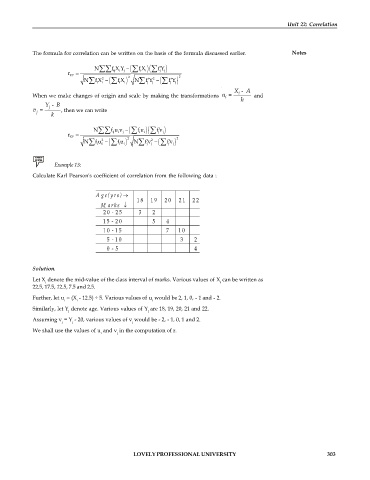
The formula for correlation can be written on the basis of the formula discussed earlier. Notes

N  f X Y   f X i  f Y  j j 
i
ij
i
j
r XY  2
2
N  f X   f X i  2 N  f Y  j j 2   f Y  j j 
i
i
i
X - A
i
When we make changes of origin and scale by making the transformations u = and
i
h
Y - B
j
v = , then we can write
j
k
N  f u v   f u  f v  
r  ij i j i i j j
XY 2 2
2
N  f u   f u i  N  f v  j 2 j   f v  j j 
i
i
i
Example 13:
Calculate Karl Pearson's coefficient of correlation from the following data :
Age(yrs) 
M arks B 18 19 20 21 22
20 - 25 3 2
15 - 20 5 4
10 - 15 7 10
5 - 10 3 2
0 - 5 4

Solution.
Let X denote the mid-value of the class interval of marks. Various values of X can be written as
i i
22.5, 17.5, 12.5, 7.5 and 2.5.
Further, let u = (X - 12.5) ÷ 5. Various values of u would be 2, 1, 0, - 1 and - 2.
i i i
Similarly, let Y denote age. Various values of Y are 18, 19, 20, 21 and 22.
j j
Assuming v = Y - 20, various values of v would be - 2, - 1, 0, 1 and 2.
j j j
We shall use the values of u and v in the computation of r.
i j

LOVELY PROFESSIONAL UNIVERSITY 303