Page 308 - DMTH404_STATISTICS

Statistics

Notes
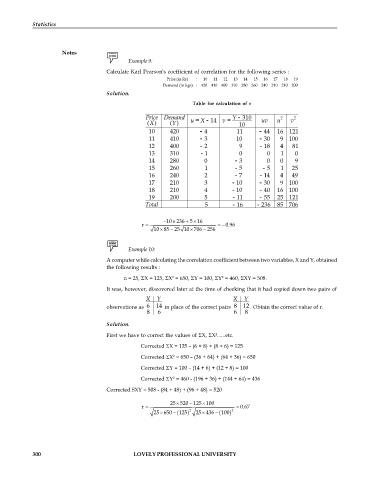
Example 9:
Calculate Karl Pearson's coefficient of correlation for the following series :
Price (in Rs) : 10 11 12 13 14 15 16 17 18 19
Demand (in kgs) : 420 410 400 310 280 260 240 210 210 200
Solution.
Table for calculation of r

Price Demand u = X - 14 v = Y - 310 2 2
(X) (Y) 10 uv u v
10 420 - 4 11 - 44 16 121
11 410 - 3 10 - 30 9 100
12 400 - 2 9 - 18 4 81
13 310 - 1 0 0 1 0
14 280 0 - 3 0 0 9
15 260 1 - 5 - 5 1 25
16 240 2 - 7 - 14 4 49
17 210 3 - 10 - 30 9 100
18 210 4 - 10 - 40 16 100
19 200 5 - 11 - 55 25 121
Total 5 - 16 - 236 85 706

 10 236 5 16



r    0.96



10 85 25 10 706 256

Example 10:
A computer while calculating the correlation coefficient between two variables, X and Y, obtained
the following results :
2
2
n = 25, X = 125, X = 650, Y = 100, Y = 460, XY = 508.
It was, however, discovered later at the time of checking that it had copied down two pairs of
X Y X Y
observations as 6 14 in place of the correct pairs 8 12 . Obtain the correct value of r.
8 6 6 8
Solution.

2
First we have to correct the values of X, X ......etc.
Corrected X = 125 – (6 + 8) + (8 + 6) = 125
2
Corrected X = 650 – (36 + 64) + (64 + 36) = 650
Corrected Y = 100 – (14 + 6) + (12 + 8) = 100

2
Corrected Y = 460 - (196 + 36) + (144 + 64) = 436
Corrected SXY = 508 - (84 + 48) + (96 + 48) = 520

25 520 125 100



r   0.67
25 650  125  2 25 436  100  2



300 LOVELY PROFESSIONAL UNIVERSITY