Page 305 - DMTH404_STATISTICS

Unit 22: Correlation

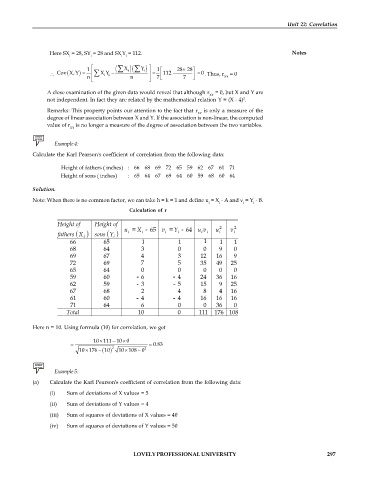
Here SX = 28, SY = 28 and SX Y = 112. Notes
i i i i

1   X i  Y  1  28 28 

i

 Cov X,Y   X Y     112    0 . Thus, r = 0
i
i
n  n  7  7  XY
 
A close examination of the given data would reveal that although r = 0, but X and Y are
XY
not independent. In fact they are related by the mathematical relation Y = (X - 4) .
2
Remarks: This property points our attention to the fact that r is only a measure of the
XY
degree of linear association between X and Y. If the association is non-linear, the computed
value of r is no longer a measure of the degree of association between the two variables.
XY
Example 4:
Calculate the Karl Pearson's coefficient of correlation from the following data:
Height of fathers ( inches) : 66 68 69 72 65 59 62 67 61 71
Height of sons ( inches) : 65 64 67 69 64 60 59 68 60 6 4

Solution.
Note: When there is no common factor, we can take h = k = 1 and define u = X - A and v = Y - B.
i i i i
Calculation of r

Height of Height of 2 2
fathers X b g sons Y b g u = X - 65 v = Y - 64 u v i u i v i
i
i
i
i
i
i
i
66 65 1 1 1 1 1
68 64 3 0 0 9 0
69 67 4 3 12 16 9
72 69 7 5 35 49 25
65 64 0 0 0 0 0
59 60 - 6 - 4 24 36 16
62 59 - 3 - 5 15 9 25
67 68 2 4 8 4 16
61 60 - 4 - 4 16 16 16
71 64 6 0 0 36 0
Total 10 0 111 176 108
Here n = 10. Using formula (10) for correlation, we get




10 111 10 0
  0.83
10
10 176    2 10 108 0 2



Example 5:
(a) Calculate the Karl Pearson's coefficient of correlation from the following data:
(i) Sum of deviations of X values = 5
(ii) Sum of deviations of Y values = 4
(iii) Sum of squares of deviations of X values = 40
(iv) Sum of squares of deviations of Y values = 50

LOVELY PROFESSIONAL UNIVERSITY 297