Page 315 - DMTH404_STATISTICS

Unit 22: Correlation

Notes
1  d 2 i
From this, we can write 1    2
n 2 X

1  d i 2 1  d 2 i 12 6 d i 2
or      1    1
1
2
n 2 2 n 2 n  1 n  n   1
2
X
Note: This formula is not applicable in case of a bivariate frequency distribution.
Example 15:
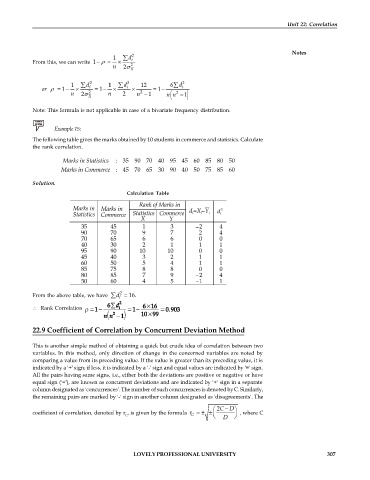
The following table gives the marks obtained by 10 students in commerce and statistics. Calculate
the rank correlation.

Marks in Statistics : 35 90 70 40 95 45 60 85 80 50
Marks in Commerce : 45 70 65 30 90 40 50 75 85 60

Solution.

Calculation Table

2
From the above table, we have  d  16.
i
2
6 d 6 16
 Rank Correlation  1 i 1 0.903
e
n n 2 1j 10 99
22.9 Coefficient of Correlation by Concurrent Deviation Method

This is another simple method of obtaining a quick but crude idea of correlation between two
variables. In this method, only direction of change in the concerned variables are noted by
comparing a value from its preceding value. If the value is greater than its preceding value, it is
indicated by a '+' sign; if less, it is indicated by a '-' sign and equal values are indicated by '=' sign.
All the pairs having same signs, i.e., either both the deviations are positive or negative or have
equal sign ('='), are known as concurrent deviations and are indicated by '+' sign in a separate
column designated as 'concurrences'. The number of such concurrences is denoted by C. Similarly,
the remaining pairs are marked by '-' sign in another column designated as 'disagreements'. The


 2 C D 
coefficient of correlation, denoted by r , is given by the formula r      , where C
C
C  D 

LOVELY PROFESSIONAL UNIVERSITY 307