Page 390 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Unit 23: Wave and Diffusion Equations by Separation of Variable

L.H.S. can be considered as the Fourier since expansion of the R.H.S. Hence Notes
2 L n x
C n f x sin dx ...(ix)
L 0 L
n c 2 L n x
and D n g x sin dx ...(x)
L L 0 L
These values completely satisfy the solution (viii). Thus u (x, t) given by (viii) with the coefficients
(iv) and (x) is the solution of the above equation that satisfies the conditions (i), (ii), (iii) and (iv).
23.1.2 Two Dimensional Wave Equation

As another example leading to the solution of the wave equation, let us consider the oscillations of
2
a flexible membrane. Let us suppose that the membrane has a density of m gms. per cm and that
it is pulled evenly around its edge with a tension of T dynes per cm. length of edge. If the
membrane is perfectly flexible, this tension will be distributed evenly throughout its area, that is,
the material on opposite sides of any line segment dx is pulled apart with a force of T dx dynes.

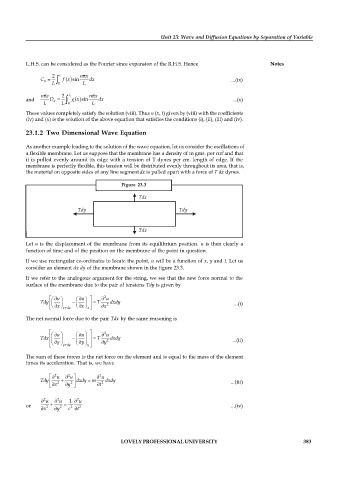
Figure 23.3

Tdx

Tdy Tdy

Tdx

Let u is the displacement of the membrane from its equilibrium position. u is then clearly a
function of time and of the position on the membrane of the point in question.

If we use rectangular co-ordinates to locate the point, u will be a function of x, y and t. Let us
consider an element dx dy of the membrane shown in the figure 23.3.
If we refer to the analogous argument for the string, we see that the new force normal to the
surface of the membrane due to the pair of tensions Tdy is given by

u u 2 u
Tdy T dxdy ...(i)
x x x 2
x dx x
The net normal force due to the pair Tdx by the same reasoning is

u u 2 u
Tdx T 2 dxdy ...(ii)
y y y
y dy y
The sum of these forces is the net force on the element and is equal to the mass of the element
times its acceleration. That is, we have

2 u 2 u 2 u
Tdy dxdy m dxdy ...(iii)
x 2 y 2 t 2

2 2 2
u u 1 u
or 2 2 2 2 ...(iv)
x y c t

LOVELY PROFESSIONAL UNIVERSITY 383