Page 391 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Differential and Integral Equation

Notes
T
where c
m
Equation (iv) is the wave equation for membrane.

Solution of Two Dimensional Wave Equation

Let us now obtain the solution of the two dimensional wave equation. In the last section we have
derived that the oscillations of a perfectly flexible membrane stretched to a uniform tension T
are governed by the two dimensional wave equation. Here in this equation u(x, y, t) is the
deflection of the membrane.
Let f(x, y) be the initial deflection and g (x, y) be the initial velocity of the membrane.
Therefore the boundary conditions and initial conditions are

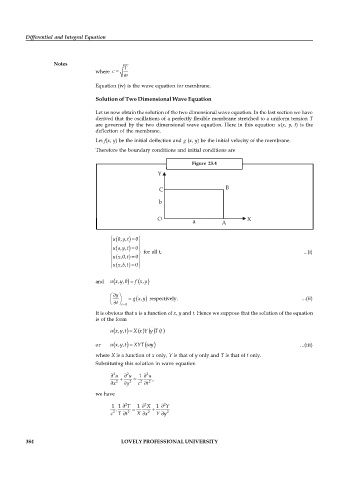
Figure 23.4

Y

C B

b

O X
a A

y
u 0, ,t 0
u a , ,t 0
y
for all t, ...(i)
u x ,0,t 0
b
u x , ,t 0
and u x , ,0 f , x y
y
y
g , x y respectively. ...(ii)
t
t 0
It is obvious that u is a function of x, y and t. Hence we suppose that the solution of the equation
is of the form
u x , ,t X x Y y T t
y
or u x , ,t XYT say ...(iii)
y
where X is a function of x only, Y is that of y only and T is that of t only.
Substituting this solution in wave equation

2 2 2
u u 1 u ,
x 2 y 2 c 2 t 2
we have
1 1 2 T 1 2 X 1 2 Y
.
c 2 T t 2 X x 2 Y y 2

384 LOVELY PROFESSIONAL UNIVERSITY