Page 48 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Unit 2: Legendre’s Polynomials

Notes
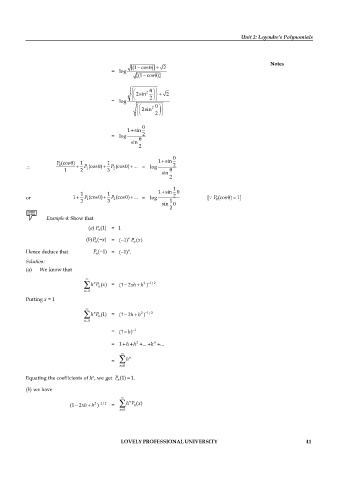
[(1 cos )] 2
= log
[(1 cos )]

2sin 2 2
2
= log
2sin 2
2

1 sin
= log 2
sin
2

P 0 (cos ) 1 P (cos ) 1 P (cos ) ... = log 1 sin 2
1 2 1 3 2
sin
2
1
1 1 1 sin 2

or 1 P 1 (cos ) P 2 (cos ) ... = log [ P 0 (cos ) 1]
2 3 1
sin
2
Example 4: Show that
( ) P n (1) = 1
a
n
b
( )P n ( x ) = ( 1) P n ( )
x
n
Hence deduce that P n ( 1) = ( 1) .
Solution:
(a) We know that

n
x
h P n ( ) = (1 2xh h 2 ) 1/2
n 0
Putting x = 1
n
h P n (1) = (1 2h h 2 ) 1/2
n 0
= (1 h ) 1

= 1 h h 2 ... h n ...

= h n
n 0
n
Equating the coefficients of h , we get P n (1) 1.
(b) we have

n
x
(1 2xh h 2 ) 1/2 = h P n ( )
n 0

LOVELY PROFESSIONAL UNIVERSITY 41