Page 86 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Unit 4: Laguerre Polynomials

Notes
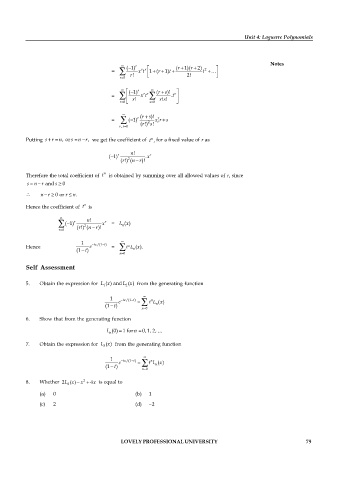
( 1) r r r (r 1)(r 2) 2
= x t 1 (r 1)t t
! r 2!
r 0
( 1) r r r (r s )! s
= x t .t
! r r ! !
s
r 0 s 0
(r s )! r
r
= ( 1) 2 x r s
t
( !) !
r
s
, r s 0
Putting s r n , or s n , r we get the coefficient of t n , for a fixed value of r as
! n
( 1) r .x r
2
( !) (n r )!
r
n
Therefore the total coefficient of t is obtained by summing over all allowed values of r, since
s n r and s 0
n r 0 or r . n

n
Hence the coefficient of t is
n ! n
( 1) r 2 x r = L n ( )
x
r
( !) (n r )!
r 0
1 tx /(1 t )
x
Hence e = t L n ( ).
n
(1 t )
n 0
Self Assessment
5. Obtain the expression for L 1 ( ) and ( ) from the generating function
x
L
x
2
1 tx /(1 t ) n
e t L n ( )
x
(1 t )
n 0
6. Show that from the generating function
L n (0) 1 for n 0, 1, 2,
x
7. Obtain the expression for L 3 ( ) from the generating function
1 tx /(1 t ) n
x
e t L ( )
(1 t ) n
n 0
8. Whether 2L 2 ( ) x 2 4x is equal to
x
(a) 0 (b) 1
(c) 2 (d) 2

LOVELY PROFESSIONAL UNIVERSITY 79