Page 91 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Differential and Integral Equation

Notes
n
n
x
x
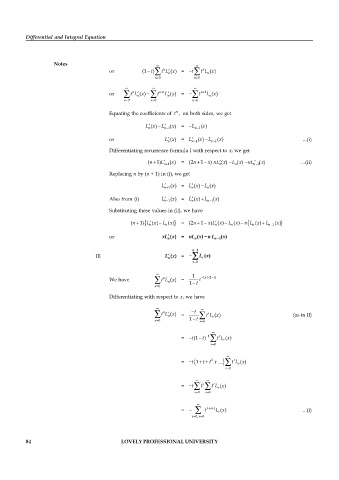
or (1 t ) t L n ( ) = t t L n ( )
n 0 n 0
n
x
or t L n ( ) t n 1 L n ( ) = t n 1 L n ( )
x
x
n 0 n 0 n 0
Equating the coefficients of t n , on both sides, we get
x
x
L n ( ) L n 1 ( ) = L n 1 ( )
x
x
x
x
or L n ( ) = L n 1 ( ) L n 1 ( ) ...(i)
Differentiating recurrence formula I with respect to x, we get
x
x
(n 1)L ( ) = (2n 1 x nL ( ) L ( ) nL ( ) ...(ii)
x
x
)
n 1 n n n 1
Replacing n by (n + 1) in (i), we get
x
x
L ( ) = L ( ) L ( )
x
n 1 n n
x
x
x
Also from (i) L n 1 ( ) = L n ( ) L n 1 ( )
Substituting these values in (ii), we have
L
(n 1) L n ( ) L n ( ) = (2n 1 x ) ( ) L n ( ) n L n ( ) L n 1 ( )
x
x
x
x
x
x
n
x
x
x
or xL n ( ) = nL n ( ) n L n 1 ( )
n 1
x
x
III L n ( ) = L r ( )
r 0
1 tx /(1 )
t
n
We have t L ( ) = e
x
n 1 t
n 0
Differentiating with respect to x, we have
n
x
t L ( ) = t r
x
n t L r ( ) (as in II)
n 0 1 t r 0
r
x
= t (1 t ) 1 t L ( )
r
r 0
r
x
= t 1 t t 2 t L r ( )
r 0
r
x
= t t s t L ( )
r
s 0 r 0
x
= t r s 1 L r ( ) ...(i)
s 0, r 0
84 LOVELY PROFESSIONAL UNIVERSITY