Page 90 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Unit 4: Laguerre Polynomials

Notes
x
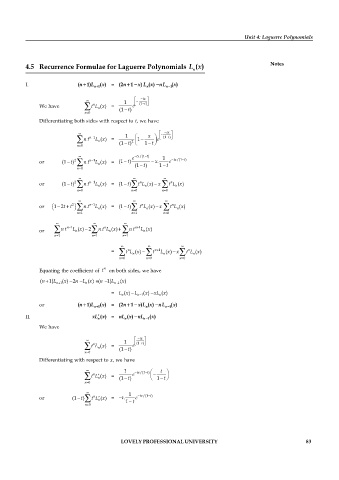
4.5 Recurrence Formulae for Laguerre Polynomials L ( )
n
x
x
)
x
I. (n 1)L n 1 ( ) = (2n 1 x L n ( ) nL n 1 ( )
tx
1 (1 t )
n
x
We have t L n ( ) = e
n 0 (1 t )
Differentiating both sides with respect to t, we have
tx
n t n 1 L n ( ) = 1 2 1 x e (1 t )
x
n 0 (1 t ) 1 t
e tx /(1 ) t 1 tx /(1 )
t
1
n
2
x
or (1 t ) n t L n ( ) = (1 t ) . x e
n 0 (1 t ) 1 t
n
n
or (1 t ) 2 n t n 1 L ( ) = (1 t ) t L ( ) x t L ( )
x
x
x
n n n
n 0 n 0 n 0
n
n
or 1 2t t 2 n t n 1 L n ( ) = (1 t ) t L n ( ) x t L n ( )
x
x
x
n 1 n 1 n 0
n
x
x
x
or n t n 1 L n ( ) 2 n t L n ( ) n t n 1 L n ( )
n 1 n 1 n 1
n
n
x
= t L n ( ) t n 1 L n ( ) x t L n ( )
x
x
n 0 n 0 n 0
n
Equating the coefficient of t on both sides, we have
x
(n 1)L n 1 ( ) 2n L n ( ) (n 1)L n 1 ( )
x
x
x
= L n ( ) L n 1 ( ) xL n ( )
x
x
x
x
or (n 1)L n 1 ( ) = (2n 1 x )L n ( ) nL n 1 ( )
x
x
x
x
II. xL n ( ) = nL n ( ) nL n 1 ( )
We have
tx
1 (1 t )
n
x
t L n ( ) = e
n 0 (1 t )
Differentiating with respect to x, we have
1 tx /(1 t ) t
n
x
t L n ( ) = (1 t ) e 1 t
n 0
1 tx /(1 t )
n
x
or (1 t ) t L n ( ) = . t e
n 0 1 t
LOVELY PROFESSIONAL UNIVERSITY 83