Page 155 - DMTH505_MEASURE_THEOREY_AND_FUNCTIONAL_ANALYSIS

Measure Theory and Functional Analysis

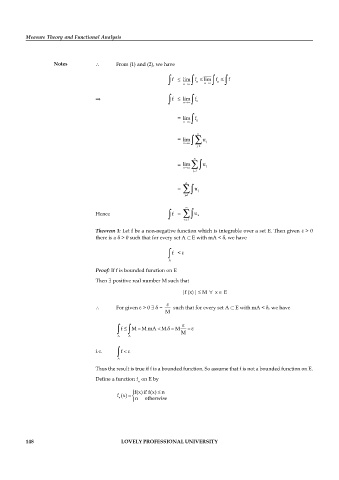
Notes From (1) and (2), we have

f lim f lim f f
n n
n n
f lim f
n n

= lim f
n n
n
= lim u j
n
j 1
n
= lim u j
n
j 1
n
= u j
j 1

Hence f = u n
n 1
Theorem 3: Let f be a non-negative function which is integrable over a set E. Then given > 0
there is a > 0 such that for every set A E with mA < , we have

f <
A
Proof: If f is bounded function on E
Then positive real number M such that

|f (x)| M x E

For given > 0 = such that for every set A E with mA < , we have
M

f M M mA M. M
M
A A
i.e. f
A
Thus the result is true if f is a bounded function. So assume that f is not a bounded function on E.

Define a function f on E by
n
f(x) if f(x) n
f (x)
n n otherwise

148 LOVELY PROFESSIONAL UNIVERSITY