Page 159 - DMTH505_MEASURE_THEOREY_AND_FUNCTIONAL_ANALYSIS

Measure Theory and Functional Analysis

Notes
= f
E i
i 1 E

= f
i 1 E i

nx
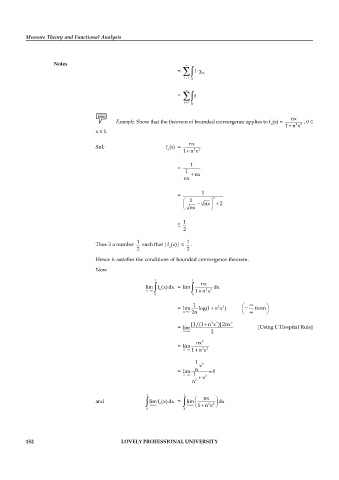
Example: Show that the theorem of bounded convergence applies to f (x) = , 0
n 2 2
1 n x
x 1.
nx
Sol: f (x) =
2
n 1 n x 2
1
=
1
nx
nx
1
= 2
1
nx 2
nx
1
2
1 1
Thus a number such that |f (x)| .
n
2 2
Hence it satisfies the conditions of bounded convergence theorem.

Now
1 1
nx
lim f (x) dx = lim dx
2
n n 1 n x 2
0 0
1
2
2
= lim log(1 n x )  form
n 2n
2
2
[1/(1 n x )] 2nx 2
= lim [Using L’Hospital Rule]
n 2
nx 2
= lim
2
n 1 n x 2
1 2
x
= lim n 0
n 1 2
x
n 2
1 1
nx
and limf (x) dx = lim dx
n
2
n n 1 n x 2
0 0

152 LOVELY PROFESSIONAL UNIVERSITY