Page 163 - DMTH505_MEASURE_THEOREY_AND_FUNCTIONAL_ANALYSIS

Measure Theory and Functional Analysis

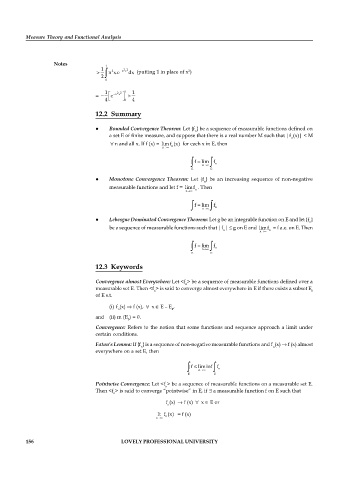
Notes 1
1 2 n x 2
2 2
n x.e dx (putting 1 in place of x )
2
0
1 n x 1 1
2 2
= e
4 0 4
12.2 Summary

 Bounded Convergence Theorem: Let {f } be a sequence of measurable functions defined on
n
a set E of finite measure, and suppose that there is a real number M such that |f (x)| < M
n
n and all x. If f (x) = lim f (x) for each x in E, then
n n
f lim f
n n
E E
 Monotone Convergence Theorem: Let {f } be an increasing sequence of non-negative
n
measurable functions and let f = lim f . Then
n n
f lim f
n n
 Lebesgue Dominated Convergence Theorem: Let g be an integrable function on E and let {f }
n
be a sequence of measurable functions such that |f | g on E and lim f = f a.e. on E. Then
n n
n
f lim f n
n
E E

12.3 Keywords

Convergence almost Everywhere: Let <f > be a sequence of measurable functions defined over a
n
measurable set E. Then <f > is said to converge almost everywhere in E if there exists a subset E
n 0
of E s.t.
(i) f (x) f (x), x E – E ,
n 0
and (ii) m (E ) = 0.
0
Convergence: Refers to the notion that some functions and sequence approach a limit under
certain conditions.
Fatou’s Lemma: If {f } is a sequence of non-negative measurable functions and f (x) f (x) almost
n n
everywhere on a set E, then

f liminf f n
n
E E
Pointwise Convergence: Let <f > be a sequence of measurable functions on a measurable set E.
n
Then <f > is said to converge “pointwise” in E, if a measurable function f on E such that
n
f (x) f (x) x E or
n
lt f (x) = f (x)
n n

156 LOVELY PROFESSIONAL UNIVERSITY