Page 76 - DCOM303_DMGT504_OPERATION_RESEARCH

Unit 3: Linear Programming Problem – Simplex Method

We can answer questions such as, Notes
1. How will a change in an objective function coefficient affect the optimal solution?
2. How will a change in a right-hand side value for a constraint affect the optimal solution?
For example, a company produces two products x and x with the use of three different materials
1 2
1, 2 and 3. The availability of materials 1, 2 and 3 are 175, 50 and 150 respectively. The profit for
selling per unit of product x is ` 40 and that of x is ` 30. The raw material requirements for the
1 2
products are shown by equations, as given below.
Z = 40x + 30x
max 1 2
Subject to constraints

4x + 5x  175 (a)
1 2
2x  50 (b)
2
6x + 3x  150 (c)
1 2
where x , x  0
1 2
The optimal solution is
x = ` 12.50
1
x = ` 25.00
2
Z = 40 × 12.50 + 30 × 25.00
max
= ` 1250.00
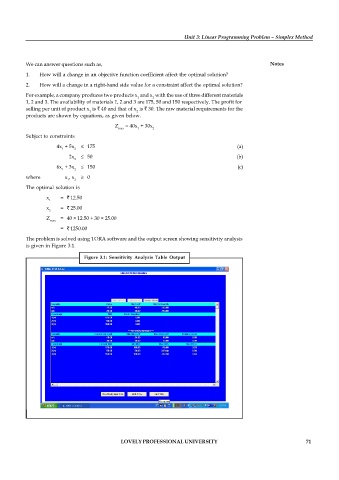
The problem is solved using TORA software and the output screen showing sensitivity analysis
is given in Figure 3.1.

Figure 3.1: Sensitivity Analysis Table Output

LOVELY PROFESSIONAL UNIVERSITY 71