Page 157 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 10: Correlation: Scatter Diagram Method, Karl Pearson's Coefficient of Correlation

correlation describes not only the magnitude of correlation but also its direction. Thus, + .8 would Notes
mean that correlation is positive because the signs of r is + and the magnitude of correlation is .8.
The above formula for computing Pearsonian coefficient of correlation can be transformed in the
following form which is easier to apply:
∑ xy
r = ... (ii)
∑ x × ∑ y 2
2

where x = ( − ) XX and y = ( − ) YY .
It is obvious that while applying this formula we have not to calculate separately the standard deviation
of X and Y series as is necessary while applying formula (i). This simplifies greatly the task of calculating
correlation coefficient.
Steps

(i) Take the deviation of X series from the mean of X and denote the deviations by x.
2
(ii) Square these deviations and obtain the total, i.e., ∑x .
(iii) Take the deviations of Y series from the mean of Y and denote these deviations by y.
2
(iv) Square these deviations and obtain the total, i.e., ∑y .
(v) Multiply the deviation of X and Y series and obtain the total, i.e., ∑xy .

2
2
(vi) Substitute the values of ∑xy , ∑x and ∑y in the above formula.
The following examples will illustrate the procedure:
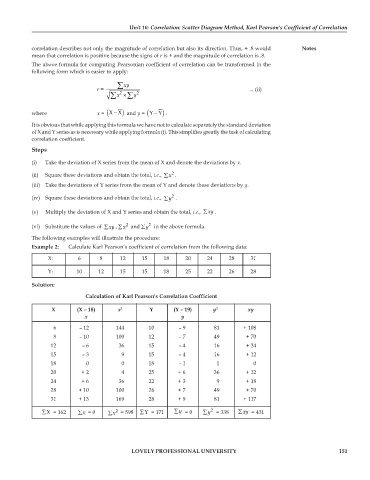
Example 2: Calculate Karl Pearson’s coefficient of correlation from the following data:

X: 6 8 12 15 18 20 24 28 31
Y: 10 12 15 15 18 25 22 26 28

Solution:
Calculation of Karl Pearson's Correlation Coefficient

X (X – 18) x 2 Y (Y – 19) y 2 xy
x y
6 – 12 144 10 – 9 81 + 108
8 – 10 100 12 – 7 49 + 70
12 – 6 36 15 – 4 16 + 24
15 – 3 9 15 – 4 16 + 12
18 0 0 18 – 1 1 0
20 + 2 4 25 + 6 36 + 12
24 + 6 36 22 + 3 9 + 18
28 + 10 100 26 + 7 49 + 70
31 + 13 169 28 + 9 81 + 117
2
2
∑X = 162 ∑x = 0 ∑x = 598 ∑Y = 171 ∑y = 0 ∑y = 338 ∑xy = 431

LOVELY PROFESSIONAL UNIVERSITY 151